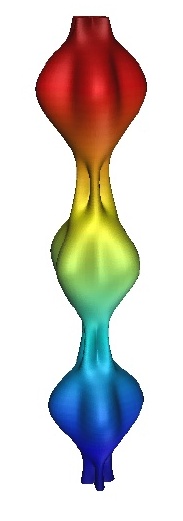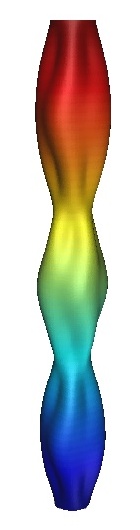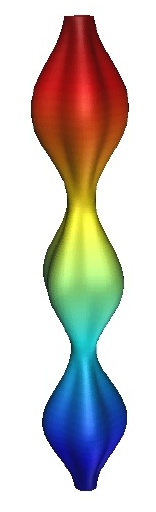|
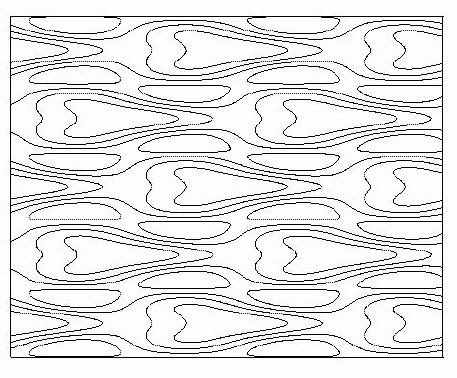
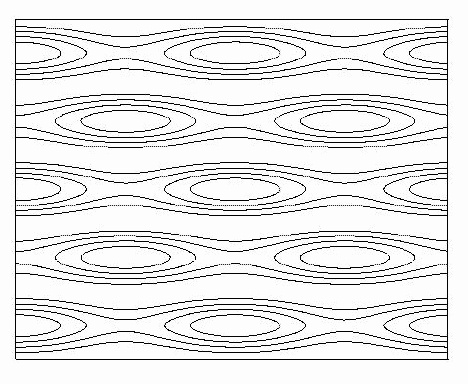
Next: Columns with Finite Symmetry Up: Columns with Discrete Symmetry Previous: Periodic Columns with No Discrete Corkscrew Columns -- Five TypesThere are three column types having
There are two continuous families depending on
Next: Columns with Finite Symmetry Up: Columns with Discrete Symmetry Previous: Periodic Columns with No Marty Golubitsky 2001-01-29 |