Introduction

The Yale art historian George Hersey showed us the columns in Figure 1 and asked us whether the ideas of symmetry breaking could be used to help classify architectural columns. Provoked by this question and the intriguing columns in that figure, we attempted to answer Hersey in the following way. We view a column as a deformed cylinder and column symmetries as the subgroup of the symmetries of the cylinder that preserve the column.

  

Figure: Plate xv from G. Guarini [2]. (Left) Fluted column (see Figure 7). (Right) Spiral column with k large (see Figure 9). (Center) Spiral column with k=1. See Table 2 for a definition of k.

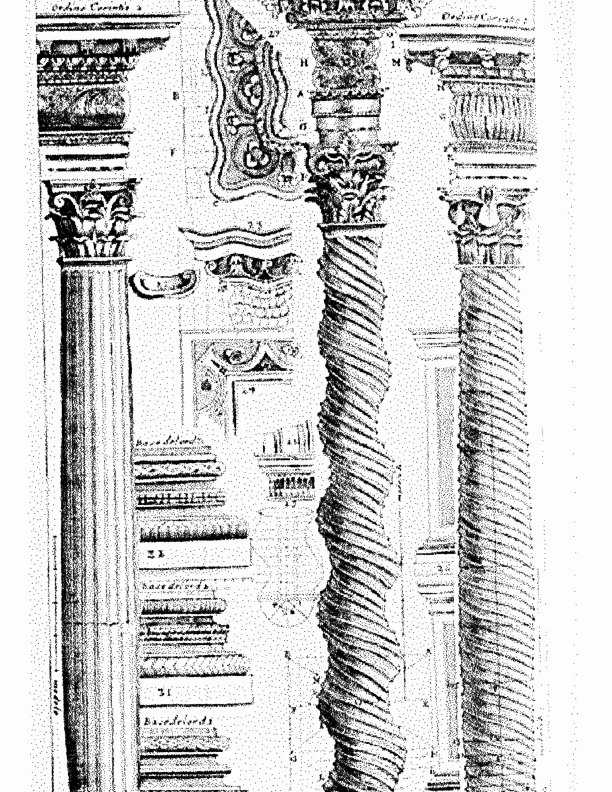
More precisely, we think of a column as a function on a cylinder (either finite or infinite) where the function tells us how far to deform the cylinder in the direction normal to the cylinder. The symmetries of a column are the symmetries that preserve the level contours of the function, that is, the isotropy subgroup of the defining function. In this paper we present the mathematical classification of the 29 different types of column symmetry. We note that there is a related classification of the rod groups that corresponds to the columns with discrete symmetry. See [1]. The classification theorem is stated and proved in Section 2. Level contours (drawn on a flattened cylinder using MATLAB) of representatives of the twenty-eight nontrivial column symmetry types are presented in Section 3, as are three-dimensional images of columns drawn using geomview.