3.2 The Symmetry Group of Friezes

Definition 3.2.1. A subgroup B of D_E2 is the symmetry group of friezes (abbreviated: the frieze group) if it's translational subgroup is generated by one translation.

The name originated from the friezes occurring in art [6, 8], the decorations obtained by repeating one chosen, symmetric or asymmetric motif. The elements of frieze groups are called the symmetries of friezes.

In further text frieze groups will be denoted as B, and their corresponding translational subgroup and point group as T_B and O_B, respectively.

If the translation by a vector a generates T_B then the lattice is the set of points R = {na | n Î Z}.

By _q will be denoted the matrix of rotation about the origin through the angle q, and by S_f the reflection in the line passing through the origin of slope f/2.

In the classification of the frieze groups, the vector a will be considered as collinear to the x-axis. Now we have that every point of the lattice belongs to x-axis. So, there hold:

Theorem 3.2.1. The point group of every frieze group B must leave x-axis invariant.

Lemma 3.2.1. Only possible transformations contained in the orthogonal group O_B are: I, -I, S₀ and S_[(p)/2].

By choosing possible orthogonal groups for the frieze groups, respecting the condition that it must leave the lattice invariant (Theorem 3.1) we will demonstrate that there are 7 non-isomorphic frieze groups.

We adopt here the two-symbol notation for friezes [6], which consists of:

- a letter m at the first position if the group contains the reflection in the vertical axis; otherwise, we have 1 on the first position;

- the second symbol is: m if the group contains the reflection in the horizontal line; g if the glide reflection belongs to the group and the reflection in the horizontal axis does not belong to the group; 2 if there is a half-turn in the group and no glide reflections; in case that none of these conditions is satisfied on the second position.

With regard to O_B, we have the following possibilities:

(1) O_B = { I} .

B = T_B

This is the frieze group 11. The orthogonal group is trivial.

(2) O_B = á-I ñ{ I,-I}

B = T_BÈT_B(0,-I)

This is the frieze group 12. The orthogonal group is C₂.

(3) O_B = áS₀ ñ{ I,S₀}. Let S₀ be realized as (aa,S₀).

(a) In the case if S₀ is realized as a reflection:

B = T_BÈT_B(0,S₀)

This is the frieze group 1m.

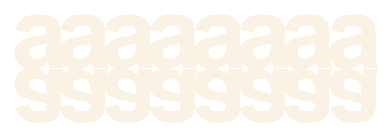(b) The other possibility is: the frieze group does not contain S₀, (0,S₀) Ï B. Then a Ï Z. Since (aa,S₀)² = (2aa,I), we have that a = n+¹/₂, n Î Z. So, S₀ is realized as a glide reflection:

B = T_BÈT_B(¹/₂a,S₀)

This is the frieze group 1g.

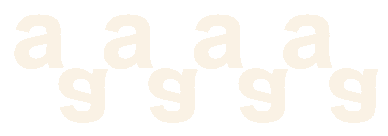The orthogonal group of 1m and 1g is D₁.

(4) O_B = áS_{[(p)/ 2]} ñ{I, S_{[(p)/ 2]},}. The S_{[(p)/ 2]} in B must be realized as a reflection. In B there is no translation by a vector normal to x-axis. Hence:

B = T_BÈT_B(0,S_{[(p)/ 2]})

This is the frieze group m1. The orthogonal group is D₁.

(5) O_B = áS₀,S_{[(p)/ 2]} ñ{ I,-I,S₀,S_{[(p)/ 2]}}. There are two possibilities:

(a) S₀ is realized as a reflection:

B = T_BÈT_B(0,-I)ÈT_B(0,S₀)ÈT_B(0,S_{[(p)/ 2]})

This is the frieze group mm.

(b) S₀ is not realized as a reflection. Then, from the same reason as in (3(b)), S₀ in B is realized as ((n+¹/₂)a,S₀), so we have that -I is realized as ((n+¹/₂)a,S₀)(0,S_{[(p)/ 2]}) = ((n+¹/₂)a,-I).

B = T_BÈT_B(0,-I)ÈT_B(¹/₂a,S₀)ÈT_B(0,S_{[(p)/ 2]})

We obtain the frieze group mg.

The orthogonal groups of mm and mg are isomorphic to D₁×D₁.

For completing the classification of frieze groups, we need to show that among the 7 groups derived there are no mutually isomorphic ones. For that, we need to check two classes of groups: 12, 1m, 1g, m1, or mm, mg (Theorem 3.2, Corollary 3.1). Among the groups 11, 1m, 1g, m1 only 12 contains the rotation, so it is not isomorphic to any of other groups from that class. The group 1g does not contain a reflection, so it is not isomorphic to m1 or 1m. The groups m1 and 1m are not mutually isomorphic, because 1m contains a glide reflection, while m1 does not. In the same way, we may show that for mm and mg. By proving that the frieze groups 11, 12, 1m, 1g, m1, mm and mg are all mutually non-isomorphic, we completed our classification:

Theorem 3.2.2. There are 7 different frieze groups.

The first classification of frieze groups has been done by Speiser, Pólya and Niggli in 1924 [6].