1 INTRODUCTION

Symmetrical operations often prevail in housing designs as underlying principles of their spatial composition. Their applications in housing designs are manifold. They apply to the overall housing organization as a whole. They also apply to a standard unit with its typological variations and the grouping of multiple units into a larger assemblage. When a standard unit and its variations are arranged in a mixture at a larger site, possibilities of their groupings will be considerably increased. A single housing unit may be symmetrical or asymmetrical. If a housing unit has a high level of individual symmetry, the number of ways in which it may be multiplied and grouped is usually limited. Nevertheless, if a unit is asymmetrical, the number of combinations is potentially increased and maximized. In architecture, bilateral symmetry is the most often encountered concept of symmetry in the classical period. In the twentieth century, architectural designers have explored various symmetries other than traditional bilateralism. They exploit all four types of the planar symmetry transformations–reflection, translation, rotation, and glide reflection–to building designs.
 

2 A NOTE ON SYMMETRY METHODOLOGY

Symmetries are defined as spatial transformations that maintain the congruent configuration of an object without changing its appearance as a whole. In two dimensions, there are two symmetry groups of plane symmetry: finite group and infinite group. The finite group of plane symmetry is called the point group. Spatial transformations take place in a fixed point or line. The transformations involve rotation about the point and reflection along the lines, or the combination of both. In the point symmetry group, no translation takes place. In the infinite symmetry group, spatial transformations occur where the basic movement is either translation or a glide reflection. In this group, designs, which are invariant under one directional translation, are called the frieze group, and designs under two directional translations are called the wallpaper group (March and Steadman, 1971).

In our discussion, emphasis is given to the application of point group symmetry of the regular polygon, in particular, the square, since the symmetry of the square prevails in architectural design (Weyl, 1952). There are two finite point groups in the plane: C_n (cyclic group) and D_n (dihedral group) where n represents the period of the group or the number of 360/n rotations. The number of elements in a finite group is called its order. The symmetry group of D_n has order 2n elements, while C_n has order n elements (Park, 2000). 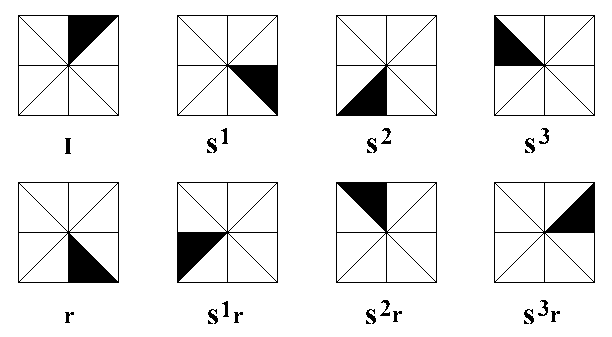The symmetry group of the square is the dihedral group D₄ of order 8. There are eight distinguishable transformations, which comprise this group (Fig. 1).
 
 

We obtain all the eight symmetry operations by combining two basic operations, quarter turn and mirror reflection. The properties construct the multiplication table of the symmetry group of the square where all possible eight transformations can be computed. Cayley diagram is another way of computing those elements where a sequence of multiplications can be tracked down. With this diagram, the symmetry group of the square can be computed to produce all of the other elements, one out of eight transformations in the case of the symmetry of the square. Equipped with the mathematical tools in mind, we analyze housing designs with regard to their use of symmetry.
 

3 F.L. WRIGHT’S QUADRUPLE BUILDING BLOCK

Frank Lloyd Wright’s social housing project, called the Quadruple Building Block, is analyzed with regard to its use of symmetry. Wright uses a standard unit plan for the project. Whereas the unit itself is asymmetric, a various local symmetries are involved in the unit. The assembly in their site layout includes two ways: One is the pinwheel type (C₄), and the other is the mirrored reflection type (D₂). Each house is set on four equally subdivided lots, sharing a common backyard in the center for all four houses. The first scheme shows a pinwheel clustering of four individual houses around the corner of a square site. Each house orients a different direction but has its own entry that faces the street. The second scheme shows each of the clustering of four houses lining both sides of the street in a mirrored format (D₂) based on the tartan grid (McCarter, 1997). The whole complex of the housings is laid out in a way that their elevation can be varied according to their arrangement. The symmetric transformation denotes differences in exterior, providing variety in the streetscape. His consecutive housing designs have been developed throughout his career, yet they share a common compositional theme: the pinwheel type of symmetry. It has been a recurring theme in his housing designs. Such a recursive theme is considered as the source of continuity of theme and variations in housing designs.
 

4 R. M. SCHINDLER’S THE SCHINDLER SHELTER

R. M. Schindler, who used work for Wright, follows Wright’s footstep in the use of symmetry. His symmetry application in housing design is highlighted in his unexecuted Schindler Shelter project, developed from 1933 to 1942. We observe that the overall floor plan is based on 5-foot square grid (with the Schell construction). Along with the square grid, the symmetry governs the internal structure of the spatial composition in each shelter unit as well as the unit variations. The internal organization is subdivided by the removable closet partitions for spatial flexibility and set along the pinwheel type of rotational symmetry (Park, 2001). Then, the garage is added to any side of the house as a separate unit. Instead of providing only a standard unit with fixed layouts, a series of variations are provided. They share the same underlying organizational system.
 

5 SUMMARY 

Symmetry as an underlying principle of housing designs has been discussed for the analysis and synthesis of housing designs. Some techniques such as multiplication table and Cayley diagram has been introduced as analytic methods. Wright and Schindler’s housing designs have been comparatively analyzed. Their use of the symmetry method in the housing designs is strikingly similar. Skylight designs are analyzed to enhance their conscious applications of the methods not only in major building designs but also in minor details.
 
 

References