Name: Judith Nem’s (Nemes), Artist (b. Budapest, Hungary, 1948). 

Address: 26bis, rue de la Varenne, F-94100 Saint-Maur des Fosses, France E-mail: judith.nemes@wanadoo.fr

Fields of interest: geometrical abstraction, minimal art, linkages between art and mathematics.

Exhibitions:Kassák Museum, Budapest (Hungary), 1992; Paris-Seoul-Tokyo-New York (traveling exhibition) 1994; Gallery Art Beam, Seoul, (Korea);Tama Art University, Tokyo (Japan), 1995; UNESCO, Paris (France); Gloria Art, Budapest (Hungary), 1997; M?vészetek Háza, Pécs, 2002. 

Abstract: The early results of a visual research programme are presented with a view towards establishing a visual language based on permutation, repetition and various mappings. The long term objective of the programme is to generate new aesthetical forms based on using elementary mathematical operations, such as decomposition, ordering and scale changes on visual objects. The paper presents some examples to illustrate the main points.
 
 

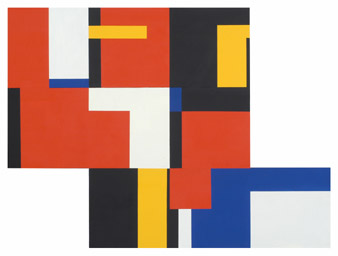
1 VISUAL PERMUTATION

One could argue that geometric art is as old as humanity itself. The history of geometric art certainly goes back to paleo times and was perhaps the earliest expression of human reflection. Its early golden age occurred as early as 1000 B.C. in early Greek art. Ever since geometric art has evolved in parallel with representational art. Whether one talks about Islamic art in general or Malevich’s Black Square in particular various forms of geometric art have always been present in the human endeavour. 

The early stage of establishing the programme was devoted to analyze the aesthetical effects of random permutations of a visual object decomposed by using a very simple rule. An earlier geometric painting of the author was used as a starting point. The painting was sub-divided into nine equidistant sub-fields that were than subjected to random permutations. The sub-fields are considered as visual letters thus every permutation yields a different visual word. The large number of 9! permutations offered surprisingly different shapes that resulted in entirely new images/words with sharply different aesthetical messages. This was particularly enhanced when different colour palettes were applied.

Figures 1 and 2 display two examples of random permutations by using different palettes.
 
 

If arranged sequentially, such as for instance could be the case of an art show, the images/words arranged define a visual sentence. As in the case of any language changing the order of words results in different meaning and visual message. Using the same visual alphabet visual words that may seem similar are constructed. 
 
 

One needs to realize, however, that this, as in any similarity, is a continuous measure of imperfect symmetry (Shu-Kun Lin, 2001).
 
 

2 SYMMETRY
 

Given that symmetry is a measure of indistiguishability the question arose what happens if visual words are doubled such as seen in Fig. 4.

The doubling has certainly contributed in a great deal to visual stability so in that regard symmetry is beautiful. However, as no new information was provided by doubling the same symmetry is ugly (op. cit.) as no new information was provided with it.
 
 

3 SEQUENCES

The random permutations as words could also be arranged into repetitive sequences yielding entirely different visual messages. Figures 5 and 6 illustrate the first two elements of such repetitive sequences. Figure 5 shows a pattern that for the very nature of repetition is a symmetrical form in itself, while Fig. 6 illustrates the case of using different visual words in a similar horizontal pattern.

[Part 2 of this paper follows with references.]