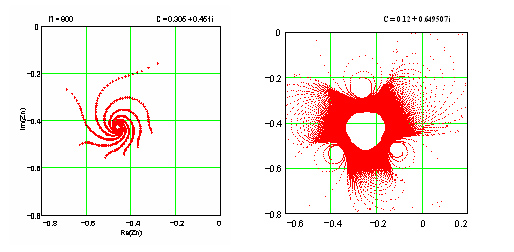
Consecutive iteration of the Mandelbrot-equation creates curious and surprising formations on the plane of the complex numbers. Here you can see two of them: from the point of  C = -0.305 + 0.451i  originates a seven-armed maelstrom, but for example in the point of  C= 0.12 + 0.649507i “lives” a “triaxial bubble-being”. Its symmetry - similarly to the biaxial symmetry of the true terrestrial animals - isn’t perfect, but it is clear.

The number of the bubbles forms a difficult but surprisingly regular structure. Similarly to the structure of the electron-clouds and quantum-numbers inside of the atoms, every bay can characterize by four numbers, depending on the numbers and shapes of the bubbles and the maelstroms. Between any of two bays lies no end of other bays, but the largest of them contents the sum of the number computable from the numbers of the bubbles of the original two bays.