CHROMATIC PLANE COMPOSITIONS

Abstract:  In this article we are studying the general problem of coloring a periodical plane mosaic, carried out by the action of a crystallographic group. In the first epigraph, we discover the color stains for a plane tiling by means of the conceptualization of plane chromatic composition. The symmetries of a chromatic composition are studied and, in Theorem 1, the plane periodical chromatic compositions are characterized. The second epigraph is dedicated to establishing a method of obtaining the chromatic plane compositions without being necessary that all the colors intervene in equal proportion. The main theorem of the article is the Theorem 2 which by means of the color equation characterizes the successions of subgroups of a crystallographic group which may be the isotropy color groups of a chromatic plane periodic composition. The article is illustrated with multiple examples, extracted fundamentally from the Alhambra of Granada (Spain). 

Keywords: Mosaic, Symmetry, Group, Tiling, Crystallographic Groups.
 

1  Introduction

We study the general problem of coloring a periodical plane mosaic, carried out by the action of a crystallographic group. Several authors (see [10] and [11]) have studied this under the restrictive hypothesis that all the colors appear equally proportionate on the chromatic composition and, even, that the crystallographic group used permutes, transitively, the color stains (perfect colorings). 

 
In the first epigraph, we discover the color stains for a plane tiling (not necessarily periodic) by means of the conceptualization of plane chromatic composition [8] and the characteristics of the paintbrushes by means of the continuous defined applications in the basic design complement. The symmetries of a chromatic compositions are studied and, in theorem, the plane periodical chromatic compositions are characterized, which give arise to the chromatic plane periodical mosaics. 

 
The second epigraph is dedicated to establishing a method of obtaining the chromatic plane compositions without being necessary that all the colors intervene in equal proportion. The regularity is described when we establish that the isotropy groups of the two different colors have to be comparable; in such a way that they form a succession of subgroups of the group used for the construction of the discolored mosaic. The main theorem of the article is the theorem which by means of the color equation characterizes the successions of subgroups of a crystallographic group which may be the isotropy color groups of a chromatic plane periodic composition. 

The article is illustrated with multiple examples, extracted fundamentally from the Alhambra of Granada (Spain), where we point out the last example which shows how the isotropy of the color subgroups may be neither normal nor comparable amongst themselves. However, the chromatic global isotropy always is normal, for being the complete intersection of a family of conjugated subgroups. 
 
 

2  Color Stains

Taking the term color in a wide sense which includes, apart from its own meaning, any characteristic of a tile, each tiling may be colored which allows us to represent a new dimension on the plane. In a precise way we establish: 

 
Definition 1 Let us call a trio (T,m,C) chromatic plane composition, where T is a tiling, C a set of colors and m an exhaustive application over of T on C, which we will call paintbrush. The number of colors of the chormatic plane composition will be the cardinal #(C). 

 
Really the chromatic plane composition k-color is the resulting class of equivalence from considering two paintbruses m₁: T®C₁ and m₂: T®C₂ equivalent if a biyective application f : C₁®C₂, with m₂ = f °m₁, exists, when C₁ and C has k colors. 

Everything said about chromatic plane k-color compositions is extended, in a natural way, to mosaics, obtaining mosaics of color. That is, when a chromatic plane composition has a associated tiling T whose support is all the plane S(T) = R² which will be called a mosaic of color. 

As a paintbrush colors tiles, we can associate the color of the tile to its points. This correspondence between points and colors is not one-to-one for which, in general, we do not have an application between the support, S(T), and the set of colors, but a multiform application. 

Proposition 1 In a chromatic composition points of the tiling which are colored with more than one color may exist. 

Proof: If two tiles are cut on the edge, the paintbrush will give these points the colors corresponding to such tiles, more than one of them being able to correspond to one point . 

In fact the points with more than one color, that neccesarily are in basic design, B( T), belong to the cement or plaster which join the pieces of tiles, or mark the essential pattern of the stucco or plasterwork. 

Definition 2 Let us call grey points of the mosaic of color, or of the plane chromatic composition those points of the tiling which are colored with more than one color. The grey points form part of the basic design of the tiling. 
 
 

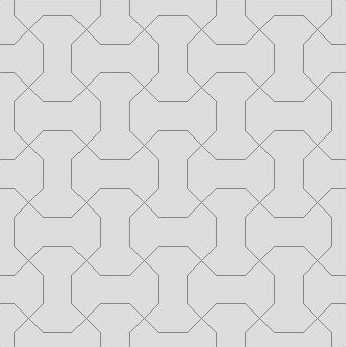
When the tiles of a tiling T have interior connexe, the following is verified. 

Proposition 2 If we consider an application m¢: S( T)-B( T) ®C, continuous and exhaustive, taking in C the discrete topology, a unique paintbrush exists m: T ®C, which assigns equal color to each point that m¢. 

Proof: As m¢ is continuous, it is constant on the connexed components of S( T)-B( T) and therefore, it is extended to a unique paintbrush m assigning the closure of each connexe component (tile of T) the constant color assigned by m¢ to the same. 

It still has to be determined how a chromatic plane composition can be enlarged mathematically to obtain a mosaic of color. To avoid problems of indetermination, we need the composition to be big enough for the rest of the mosaic to be obtained by translation . This is an abstraction which is necessary to speak with precision about the classification of the mosaics, such as those in the Alhambra. 

Definition 3 Given a plane chromatic composition (T,m,C), we will say that an isometry g of the plane is a symmetry of the chromatic plane composition if it verifies: 

• 1) S(g( T)ÇT) = g(S( T)) ÇS( T) ¹Æ. 
• 2) For each tile K of g( T) ÇT, m(K) = m(g^-1(K)).

Definition 4 The set of symmetries of the chromatic plane composition generates a subgroup of the group of isometries of the Euclid plane, that we will note Sim(T,m,C) and we will call it symmetry group associated to the chromatic plane composition, which in turn contains a normal subgroup formed by its translations, that we note T(T,m,C). 

 
The above-mentioned definitions are extended, only by substituting the term chromatic plane composition, to the mosaics of color resulting in the corresponding symmetry groups. 

 
Note that if (T,m,C) is a mosaic of color and g one of its symmetries, the condition 1) in Definition 3 would be expressed as: 

given that being S(T) = R², g(S(T)) ÇS( T) = g(R²) ÇR² = R² for which S(g(T)ÇT) = R² = S(T); having g(T) ÇT and T equal support and being the first set of tiles contained in the second, it results that g(T) ÇT = T, for which g(T) ÉT. Besides, S(g(T)) = S(T) = R². 

On the other hand, as g is an isometry it results in that the g(T) tiles are only cut on their edge, for which g(T) cannot contain more tiles than T if there were a tile in g(T) that were not in T, it would cut some tile of T in its interior. Then g(T) = T. 

As a consequence of the before-mentioned, the condition 2) in Definition 3 is expressed as: 

Therefore, the symmetries of a mosaic of color are plane isometries that, apart from transforming the mosaic in itself, keep the color. 

Definition 5 We will say a plane chromatic composition is periodic if T(T,m,C) is generated by two independent translations. 

Let us call M(T) the set of tiles È{t(T) / t ÎT(T,m,C)}. 

We announced [8] the following theorem and now we present completely its demonstration. 

Theorem 1 Let (T,m,C) be a chromatic, plane, and periodic composition. It is verified: 

a) M(T) is a mosaic.

b) A unique paintbrush, m¢: M(T) ®C, exists such that for each K of T and t ÎT(T,m,C) it is verified that m¢(t(K)) = m(K).

c) Sim(M(T),m¢,C) = Sim(T,m,C).

d) Sim(M(T),m¢,C) is a plane crystallographic group.

Proof: a) As the translations of T(T,m,C) are isometries, M(T) is a set of tiles. Let A₁, A₂ÎM(T) and pÎA₁ÇA₂, A₁ and A₂ are images by movements of tiles K₁, K₂ of T, translations which are obtained by successive compositions of symmetries of the chromatic plane composition (T,m,C). 

 
The condition 1) of the Definition 3 allows K₁ and K₂ to be chosen in a way that p is the image of a point p¢ that is in K₁ÇK₂. If p¢ is in the interior of one of those tiles, it results that K₁ = K₂ and as a consequence A₁ = A₂. On the contrary, if p¢ is in the intersection of the boundaries of K₁ and K₂, it results that p is in the intersection of the boundaries of A₁ and A₂. Therefore if two different tiles of M(T) cut themselves, they do so along the length of their boundaries. Besides, because of the Definition 3 the support of M(T) is all R². Then M(T) is a mosaic. 

 
b) In this case it is only necessary to demonstrate that the application 

being t Î T(T,m,C) and K Î T, is well-defined, then, by construction the elements of M(T) are of the form t(K). 

For that let us suppose that a tile of M(T) has two representations t₁(K₁) = t₂(K₂), K₁, K₂ÎT, t₁, t₂ÎT(T,m,C); we have that K₁ = t^-1₁(t₂(K₂)). t^-1₁° t₂ is the result of successively composing symmetries of (T,m,C) and inverse transformations of symmetries. The condition 2) of the Definition 3 guarantees that all keep the color, then m(K₁) = m(K₂). The uniqueness of m¢ is guaranteed by the construction and by the fact that m¢|_T = m. 

c) In the demonstration of this paragraph we will make use of the observations made after the Definition 3. The symmetries of a mosaic of color, (T,m,C), are the isometries of the plane which verify g(T) = T and m(T) = m(g^-1(T)). 

As both conditions are closed (stable for the inverse and composition) it results that, calling Iso(R²) the group of isometries of R², then Sim(M(T),m¢,C) = {g Î Iso(R²) / g(M(T)) = M(T) and m¢(M(T)) = m¢(g^-1(M(T)))}. 

Because of the construction of M(T) and of m¢ from (T,m,C), the symmetries of (T,m,C), generators of Sim(T,m,C), are in Sim(M(T),m¢,C), then Sim(T,m,C) ÍSim(M(T),m¢,C). 

By the same constructions, the elements g of Sim(M(T),m¢,C) which take the tiling T to g(T), with g(T) ÇT¹Æ are symmetries of (T,m,C), since that m¢|_T = m, and these elements generate Sim(M(T),m¢,C), then the equality is proved. 

d) Lastly, this paragraph is an immediate consequence of c) and of the fact that M(T) is constructed from T by translations. 

In effect, T(M(T),m¢,C) = T(T,m¢,C). Then T(M(T),m¢,C) is a subgroup of movements with translations in two independent directions and discrete action. As the other symmetries have to be compatible with the movements [2] it results that the group Sim(C(T),m¢,C) is a plane crystallographic group. Let us observe that T is contained in M(T) and m¢|_T = m. 

The mosaic of color (M(T),m¢,C) is the mathematical result of extending the chromatic plane periodic composition (T,m,C) to all the plane. 

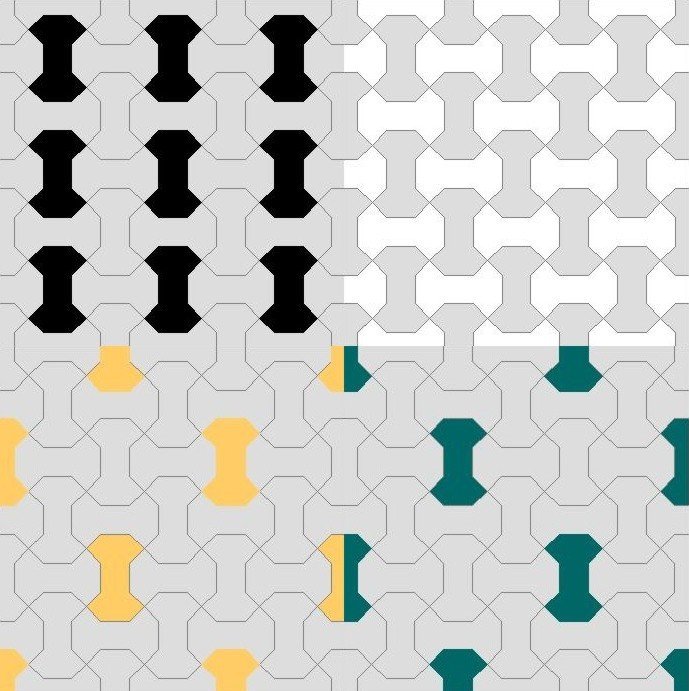
Definition 6 Let (T,m,C) be a plane chromatic composition or a mosaic of color for each cÎC, we will call a color stain the set of the plane M_c = S(m^-1(c)). Each color stain may identify itself by its own color. 
 
 

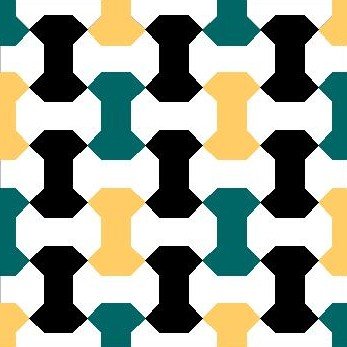
Proposition 3 The color stains are coincident or they intersect on their borders. 

Proof: Direct consequence of the paintbrush being exhaustive, of the grey points being on the edge of the tiles and of the edge of the union of subsets is contained in the union of the respective edges. 

What relationship exists between the symmetry of the color mosaic and its stains? 

We begin from a crystallographic plane group G, and let us suppose that on acting on one of its basic tiles defines a T tiling, that can be colored by a paintbrush, m, in such a way that the action of each element g, of the group G, on the tiles of T produces a paintbrush, m°g, equivalent to m. 

This condition imposed on the paintbrush is equivalent to saying that the elements of G permute the color stains of T for which the compatibility results with that studied in the previous paragraph. The permutation of colors, s_g, associated to an element g of G is given by the equation s_g(m(K)) = m(g(K)). 

As the group G acts transitively on the tiles of T, it also results that it must be able to pass from one color stain to any other, which implies that all the colors intervene in the same proportion. 

The theories that we could qualify as complete only reach interesting mathematical results when, under successive hypotheses of regularity, they practically coincide with this version. 

Example 1 Beginning from a Bone is a basic tile of a crystallographic plane group G, which could be of the p4 type, the basic design of the Polibone is obtained. The chromatic plane composition of the Alhambra counts on four colors which we can take for an application of this theory. In this way, the paintbrush, m, takes values in C = {white, honey-colored, black, green}. With the condition that G permutes these colors between themselves, one of the resulting possible chromatic compositions is that of Figure 3. This is one of the five different ones, that is, not equivalent by permutation of colors with which that group and number of colors may be constructed. 
 
 

Would the Polibone of the Throne Hall of the Alhambra be obtained by this theory? 

For the reply to be affirmative, it would be necessary to have a set C of 8 colors, since the green stains or the honey-colored ones suppose, each one of them, an eigth part of the total. To obtain a coherent result between the theory and reality, we have to abstract and think that bones of the same real color have different theoretical color, or rather, 8 paint pots exist of which four are white, two black, one green and one honey-colored. 

When the elements of G permute all the colors of a mosaic of color in itself, calling G_c the set of elements of G that leave the color stain c, M_c, invariant, it results that each class of the G/G_c quotient coincides with the equivalence class of the elements of G which determine permutations on C such that they transform c into the same color. Therefore, the class number of the quotient, index of G_c in G, coincides with the number of colors of C, and therefore with the number of stains. 

These theories have the inconvenience in that they do not give an answer to many real situations, in those which some colors are predominant over others, such as we have seen in the previous example. 

Therefore, the problem of finding a mathematical theory which interprets the periodic chromatic compositions without supposing more jumps than those really necessary remains open. The following method of coloring allows us to give a partial solution and the bringing up-to-date of tables as that given in Wieting [11] or in Santander et al. [9], in which the number of possible color groups are shown according to the number of colors which are used. 
 
 

3 Method to obtain the chromatic plane periodic compositions. 
   The color equation.

Let T_G be a tiling of the G group on R², T the subgroup of the translations of G. With T being normal, we can consider the tiling T_G/T = T\ T_G on the space Y = T\ R². 

1. We define m : T_G/TC®C as is wished. 
2. A unique paintbrush X = m°p exists such that (T_G,X,C) is a chromatic plane periodic composition. By construction we have that TÌSim(X_G,X,C). 
3. If T = Sim(T_G,X,C), the group Sim(T_G/T,m,C) is trivial and (T_G/T,m,C) does not have color symmetries. Reciprocally, if (T_G/T,m,C) does not have different symmetries of the identity, then T = Sim(T_G,X,C). 
4. T is used to extend the color of Y to all R².

If we are dealing with normal subgroups, the 2_nd theorem of isomorphy, the double quotient, allows us to obtain such an index comfortably.

Example 2 Let G₀ be a crystallographic plane group of the type p4g; G₁,G₂ and G₃ subgroups of G₀o f  the type cmm, pmm and pgg respectively. 

Let us form a succession G₀ ÉG₁É G₂ÉG₃ in which we express the index of each subgroup in the preceding group under each inclusion. Then: 

from where, #(G₀/G₃) = 2×2×4 = 16 

If we take a region unity with respect to G_i as a tile, it will be formed by a number of basic tiles with respect to G₀ equal to #(G₀/G_i). Therefore, a tile for G₀ could decompose in different ways in tiles for the subgroups G_i since #(G₀/G_n) admits the different indexes #(G₀/G_i) as dividers? This fact allows us to group them in multiples of quantities of those indexes. 

Let (T_G,X,C) be a chromatic plane periodic composition. For each color c ÎC, we consider the color stain X_c tiled by means of X^-1(c). Let G_c be the subgroup of isotropy of the color c formed by the transformations of G which leave the color c invariant. These elements are characterized by the equation X(gK) = M(K), for all K ÎX^-1(c). It is verified that Sim(T_G,X,C) ÍG_cÍ G. 

With the aim of being able to manage comfortably the family of subgroups {G_c / c ÎC}, we can suppose in what is left that all the subgroups are comparable, that is, that given the color c₁ and c₂ of C it is verified that G_c2 is a subgroup G_c1 or vice-versa. In the first case G_c2 ÍG_c1, this fact means that each element of G which leaves the color c₂ invariant also leaves the color c₁ invariant. 

When all the subgroups G_c are comparable, we can order the set of colurs C = {c₁,c₂,¼,c_n} in such a way that G_ci ÍG_ci-1, with i Î {2,¼,n}. Calling G₀ = G ans G_i = G_ci, it results in the chain of subgroups G₀ ÊG₁Ê ¼ÊG_iʼ ÊG_n. 

Note that the elements of G_n must leave all the color invariant for which its elements are symmetries of color of the chromatic composition (T_G,X,C); therefore, G_n = Sim(T_G,X,C). 

Because of the considerations made in the preceding paragraphs we will suppose that all the subgroups G_i are normal in G, being able to consider the chromatic finite composition (T_G/Gn = G_n\ T_G,m,C) with m°p = X. 

Theorem 2 If the subgroups of isotropy of the colors of a chromatic plane and periodic composition (T_G,X,C) are normal and comparable they verify: 

a) The number of tiles in G_n\ R² colored with the color c_i is equal to #(G_i/G_n).

b) #(G₀/G_n) = å_{i = 1}ⁿ  #(G_i/G_n)

c) 1/#(G₀/G₁) +¼+ 1/#(G₀/G_n) = 1

d) (¼(((#(G₀/G₁)-1)¼)#(G_i-1/G_i)-1)¼#(G_n-1/G_n)-1) = 0

Proof: a) This is a consequence of G₀/G_n acting transitive and effectively on T_G/Gn, which has #(G₀/G_n) tiles. As G_i/G_n transforms in itself the colored tiles of color c_i, of these there has to be exactly #(G_i/G_n). 

 
b) As all the tiles are colored, their number #(G₀/G_n), has to be the sum of the numbers of tiles in G_n\ R² colored with different colors. 

 
c) In G_n\ R²we obtain the tiling T_G/Gn formed by #(G₀/G_n) colored tiles. For each color c_i a stain is obtained which occupies 1/#(G₀/G_i) of the total of T_G/Gn. When i = 1,2,¼,n results it is stated. 

Also we may prove this result from 1 and b): 

equalling: 

and dividing by the first member, results: 

and applying, again, c) the paragraph results. 

d) We can transform the equation c) in this other which we will call the color equation: 

In effect, multiplying c) by #(G₀/G₁)¼#(G_n-1/G_n) and taking into account 1 have the result: 

If we repeat the procedure: 

The last step consists of: 

Passing everything to the second member d) is followed. 

The interpretation of the color equation is the following: In T_G/Gn there is #(G_i/G_n) tiles of color c_i, resulting from applying the group G_i/G_n on a tile which has not been previously painted with any of the colors c₁,¼,c_i-1. The procedure commences coloring with color c₁ the tiles which there are of this color. The step from T_G/Gn to T_G is done by means of the procedure described at the beginning of this paragraph. 

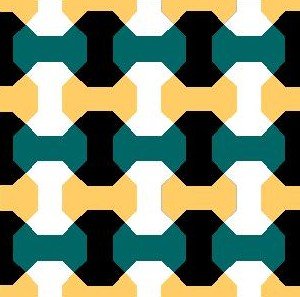
Example 3 Let us color by means of this procedure the polibone (Figure 4). Let G₀ be a crystallographic plane group of the type p4g. Let us form the chain p4g É cmm ÉpmmÉ pgg. 

In Example 2 we saw that #(p4g/pgg) = 16. 

On the other hand, 

We must extend the chain trivially: 

Therefore the number of necessary colors is 6. 

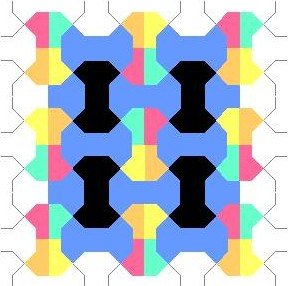
Figure 4

To conclude, we have colored in the following way: It is colored in the quotient of R² by the action of G_n in such a way that once a unit region us colored for this group, the coloring is extended to all R² by the action of G_n. In this tile, or in the quotient G_n\R², one would work with the succesion of finite conmutative groups G₀/G_nÉG₁/G_nɼÉG_n-1/G_nÉ 1 in such a way that G_n\ R² is descomposed in #(G₀/G_n) tiles, of the action of G₀/G_n on G_n\ R², and it would be in this quotient where the groups G_i/G_n would work to color. 

This version justifies the necessity of considering successions of normal subgroups, it is the only way of guaranteeing that the quotients of the groups are new groups that act in the quotient of the topological space. Besides, the problem of coloring is transformed into a finite problem of combinatory. 

The way of acting which is followed in the chromatic plane and periodic compositions consists of looking at the succession of groups from the end, instead of doing so from the beginning. The last group leaves invariant all the tiling, including the colors, while the first leaves invariant only the discolored tiling. 

This allows the general problem of coloring periodical mosaics to be reduced to that of coloring a unit region for G_n by means of a set of finite groups. 

The before-mentioned theories are contemplated through that one developed previously. To suppose that the color stains are equisuperficial, the subgroups associated to two different stains are conjugated, for which they present equal index. The chains are reduced to G = G₀ÉG₁ = G₂ = ¼ = G_n and on being 

it has to be verified that 

In this case it results that the number of colors used is #(G₀/G_n) = #(G/Sim(T_G,X,C)). 

If we take two colors, the color equation tells us that the subgroups utilized must have index 2 in the group: 

From the table of subgroups of the crystallographic plane groups [1] and if we take into account that the subgroup pmm must be counted twice, the number of dichochromatic plane groups is 46. 

However, and it is the problem that we are presently trying to resolve, there are situations in which the normality of the subgroup is not needed and, however, we can apply the method of coloring together with the color equation. Let us see how we can make the coloring of the following color mosaic, from the basic design: 
 
 

The symmetry group G associated to the basic design is of the type cmm; we take the isometries {t_a,s_H,s_L} (see Figure 6) as generators of this group. A normal subgroup G₀, of index 2 and type pmm, is generated by {t_v,t_h,s_H,s_L}, when t_v = s_Lt_as_Lt_a and t_h = s_Lt_-as_Lt_a. A subgroup not normal, of type pmm also, is the subgroup G₁ generated by {t_v,t_3h,s_H,s_L}. We form the chain of subgroups G ÉG₀ÉG₁. 
 

We colored in the following way [4]: 

1st. We take the basic tile K for G, as in the above Figure 6. 

2nd. We color the basic tile of color 1 (in reality it is black) for G and we extend this color by means of G₁. 

3rd. Again we color the basic tile t_hK of color 2 (green) and we extend this color with t_hG₁ from K. 

4th. We color of color 3 (honey-colored) and extend with t_2hG₁. 

Note that the result is different if the class G₁t_h and G₁t_2h are used in the 3rd and 4th steps. 

Now, one half of the tiles are colored. 

5th. We color again of color 0 (white) the tiles not colored above by means of subgroup G₀, starting from any tile not colored (for example, t_aK). 

In this way the mosaic of the figure is completely colored. 

The subgroup of isotropy associated to the white color is G₀. 

The subgroups of isotropy associated to the other three colors: G₁ to black, G₂ to green and G₃ to honey-color, are not normal subgroups of G and of G₀; more exactly, G₂ is generated by {t_v,t_3h,s_H,s_M}, and G₃ is generated by {t_v,t_3h,s_H,s_N}. These three subgroups are not comparable between themselves. However, they are conjugated and their intersection is the normal subgroup G¢ of the global isotropy of all colors G¢, of type pm, generated by {t_v,t_3h,s_H}: 

The subgroups of isotropy associated to the other three colors: G₁ to black, G₂ to green and G₃ to honey-color, are not normal subgroups of G and of G₀; more exactly, G₂ is generated by {t_v,t_3h,s_H,s_M}, and G₃ is generated by {t_v,t_3h,s_H,s_N}. These three subgroups are not comparable between themselves. However, they are conjugated and their intersection is the normal subgroup G¢ of the global isotropy of all colors G¢, of type pm, generated by {t_v,t_3h,s_H}: 

References:

[1]

COXETER H.S.M., Coloured Symmetry. M.C. Escher. Art and Science, North-Holland (1986), 15-33.

[2]

HILBERT D. and COHN and VOSSEN S., Geometry and the imagination, Chelsea Publishing Company, New York (1952).

[3]

PÉREZ-GÓMEZ, R., The four regular mosaics missing in The Alhambra, Comp. and Math. with Appls. 14, 2 (1987), 133-137.

[4]

PEREZ-GOMEZ, R. and RUIZ, C., Methods of perfect coloring (Visual Mathematics).

[5]

PÉREZ-GÓMEZ, R. and RUIZ, C., Tiling in Topological Spaces (to appears).

[6]

PÉREZ-GÓMEZ, R. and RUIZ, C., Kaleidoscopes in Topological Spaces (to appears).

[7]

PÉREZ-GÓMEZ, R. and RUIZ, C., Kaleidoscopes in the Alhambra, Proceeding of the IVth Workshop on Electromagnetically Induced Two-Hadron Emission(2000).

[8]

RUIZ, C. and PÉREZ-GÓMEZ, R., Visiones matemáticas de la Alhambra. El color, Epsilon 9 (1987), 51-59.

[9]

SANTANDER M., RULL F. and GALLARDO F., Clasificación de grupos de color a partir de sistemas de generadores, Bol. Soc. Española de Mineralogí a 12 (1988), 59-66.

[10]

SCHWARZENBERGER, R.L.E., Colour Symmetry, Bull. London Math. Soc. 16 (1984), 209-240.

[11]

WIETING, T.W., The mathematical theory of chomatic plane ornaments, Marcel Dekker, New York (1982).

[12]

VAN DER WAERDEN, B.L. and BURCKHARDT, J.J., Farbgruppen, Z. Kristallogr. 115 (1961), 231-234.

[13]

LUNGU, A.P. On coloring figures by different colors and their description in the framework of generalized color symmetry, (in Russian), Studies on general algebra, geometry and their applications, 104-110, 1986.

[14]

ZAMORZAEV, A.M., GALYARSKIJ, EH.I., PALISTRANT, A.F., Colour symmetry, its generalizations and applications (Tsvetnaya simmetriya, ee obobshcheniya i prilozheniya), (in Russian), Shtiintsa, Kishinev, 1978.

[15]

ZAMORZAEV, A.M., KARPOVA, Yu.S., LUNGU, A.P., PALISTRANT, A.F., P-symmetry and its further development (P-simmetriya i ee dal'nejshee razvitie), (in Russian), Shtiintsa, Kishinev, 1986.

Rafael Pérez-Gómez
Dpt. Matemática Aplicada,
EU Arquitectura Técnica,
Univ. Granada,
18071 - Granada, Spain.
e-mail: rperez@ugr.es

Ceferino Ruiz
Dpt. Geometrí a y Topologí a,
Univ. Granada,
18071- Granada, Spain.
e-mail: ruiz@ugr.es