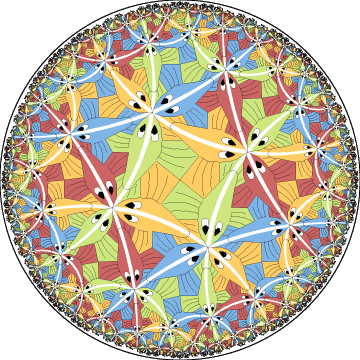
Figure 9: A rendition of Escher's
Circle Limit III pattern.
It is tempting to surmise that the white backbones form hyperbolic
lines, but this is not the case. A close examination of those lines
reveals that they make angles of approximately 80 degrees with the
bounding circle. The backbones are so-called equidistant curves,
which are represented in the Poincaré model of hyperbolic
geometry as circular arcs that are not orthogonal to the bounding
circle. All points of an equidistant curve are the same hyperbolic
distance from the hyperbolic line (orthogonal circular arc) that
has the same endpoints on the bounding circle.
Circle Limit III is also based on the tessellation {8,3}
(as is Circle Limit II). However it is more complex
since there are two kinds of 3-fold rotation points: at meeting
points of fish noses, and meeting points of left fins. Thus a
fish motif is contained in two adjacent isosceles triangles
instead of only one triangle. The computer program was not
designed to handle such a situation, but with some editing of
the motif data file it was possible to create a
Circle Limit III -
like pattern based on the tessellation {10,3}.
This pattern is shown below in
Figure 10.
It certainly requires five colors to achieve perfect color symmetry
since five fish meet at right fin tips. In fact, it turns out that
six colors are required.
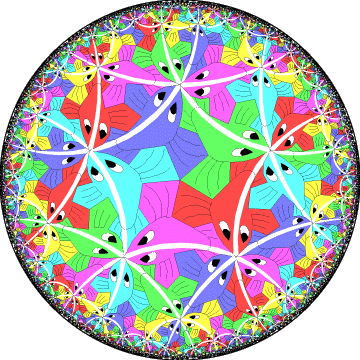
Figure 10: A pattern based on
Circle Limit III and the tessellation {10,3}.
For completeness, we show an application of the transformation
process to the Circle Limit IV pattern
(Figure 1).
As with Circle Limit II
this pattern could be transformed to a
pattern of angels and devils with any number meeting at the feet and
at the rotation points at the wing tips.
Again, such a pattern can
be constructed from the tessellation {p,q} as long as
p is even (due to the bilateral symmetry of the
angels and devils). Then p/2 angels and devils meet
foot-to-foot and q meet at the wing tips.
As shown in
Table 1,
the pattern will be hyperbolic if (p-2)(q-2) > 4.
Figure 11 below shows a
pattern of angels and devils based on the tessellation {4,5}.
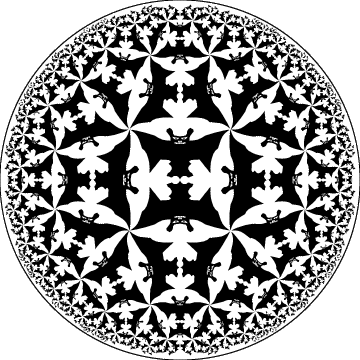
Figure 11: A pattern of angels and devils based on
Circle Limit IV and the tessellation {4,5}.