|
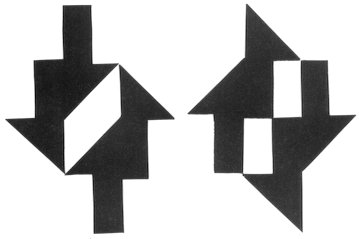
(ii) The shape of interspace Let us examine the shape of the space between units. Two situations are imaginable: the separate arrangement as in Figure 154, and the ring-shaped arrangement, circling the units which touch each other, as in Figure 156-a,b. The latter is particularly interesting as an unexpectedly simple and perfect shape can be created in its space (interspace). The two shapes of Figure 156 are the same type as that of Figure 153, for both of these are the sets of the mixed type with 4 units and take the method of (A1+A2)×2. Completely different shapes have been produced with little change in the arrangement. In Figures 155 and 157 regular grouping using A1 as the
unit creates many types of interspaces. Figures 121
and 123 on page 84 are
of the same theme. You will notice from Figures 121
and 155 that the black and white are the same in shape. This means that
when the units are stuffed skillfully, fusion occurs on all sides of the
units and the phenomenon of overall fusion in which the whole picture is
crammed with the units takes place. In other words, these units have a
complete space-filling nature like tiles (The eight examples of Figure
149 fall under this). If the issue is dealt with as that of the centripetal
KOHSEI (Basic Art & Design), it is the matter of the division
of a limited shape of two dimension space into equal shapes.
("Division and proportion", "Divisions using tiles" in Section 2 of this
chapter will take up this matter.)
|