|
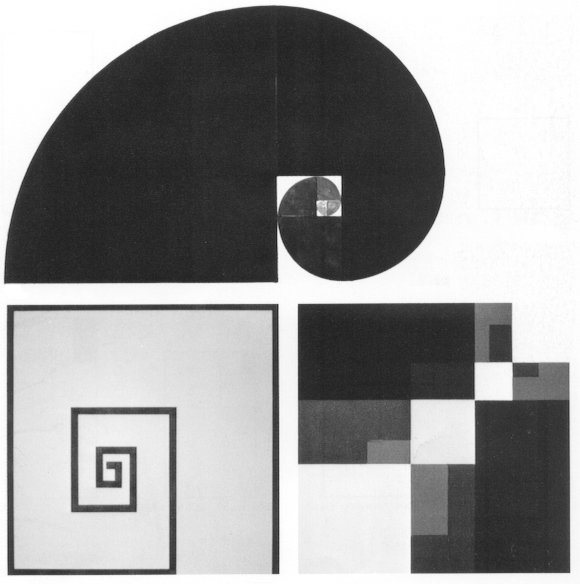
Then, because the golden ratio is a number which appears unexpectedly in various graphic drawings as seen in the example of the Pentegram, the Greek might have felt a strange charm in the ratio. In the present days, the rectangles are mostly used. But, the golden rectangles which is a little longer is still popular. These shapes look "a little thinner" or "a little longer" than the shapes seen in everyday life. This is probably the reason why they attract the sense of modern people. If a golden ratio f is shown in number, This is obtained easily if drawing is done geometrically as shown in Figure 238 though it is hard to calculate because an irrational number is contained. The midpoint E of the side AB of the square ABCD is determined at first. A circular arc with a radius of EC is drawn around E. If we assume that the intersection between the arc and the extension of the line AB is defined as F, the rectangle with the short side of AD and the long side of AF will be the golden rectangle. The following relations between the lengths of the sides are: And, as the characteristics of the golden rectangle, the close relationships
between squares should be noted. If a square whose side is the same as
the short side of the golden rectangle is removed from the golden rectangle,
the remaining shape will also be another golden rectangle (Fig. 230).
|
|||