 |
 |
The compound of three octahedra and a remarkable compound of three square dipyramids, the Escher's solid
Livio Zefiro
Dip.Te.Ris., Universita' di Genova, Italy
(E-mail address:
livio.zefiro@fastwebnet.it)
Notes
|
The Dutch artist M.C. Escher achieved a world-wide renown
for his work, covering many fields
[1]:
a particular mention should be deserved to the bidimensional periodic tessellations
of the plane by interpenetrating forms (often representing
animals, insects..), the drawing of unusual polyhedra and the reproduction of impossible objects.
Both last characteristics are present in the well-known lithograph print Waterfall
[2]
(1961), based on two Penrose triangles or "tribars", where the water, from the foot of
the waterfall, seems to return naturally to the top of the waterfall without the need to be raised.
Generally, only afterwards one notices that, in the drawing, a solid is placed on the summit of each tower
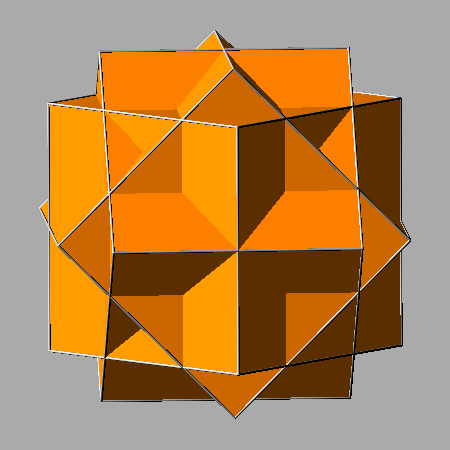
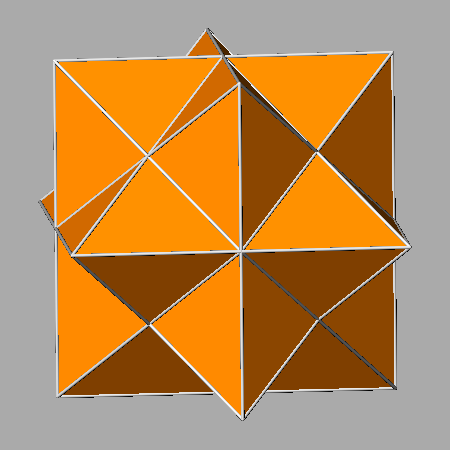
of the building: a compound of three rotated cubes on top of the left tower and
the so called Escher's solid [3] on top of the right tower.
Their remake, along the same angle of view, is shown in Fig. 1.
In this paper our attention will be focused on the Escher's
solid.
As a first step, let's try to answer to the question: which are the single
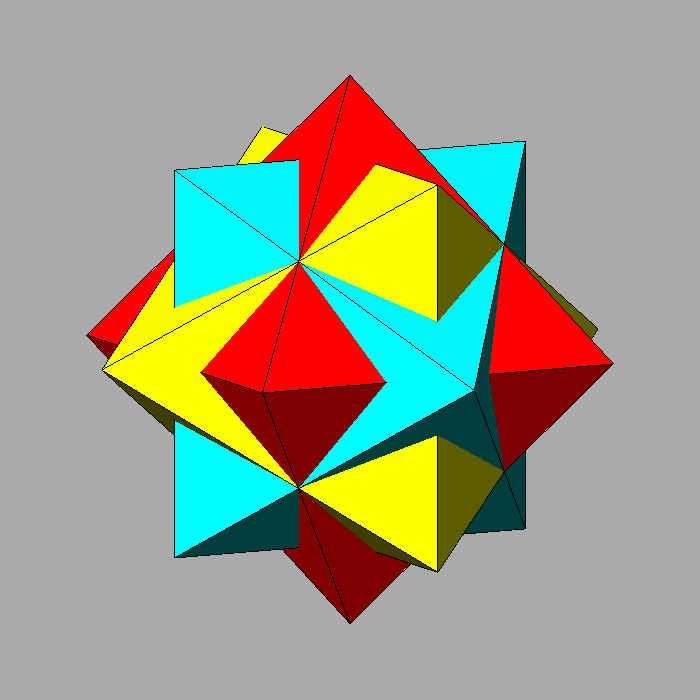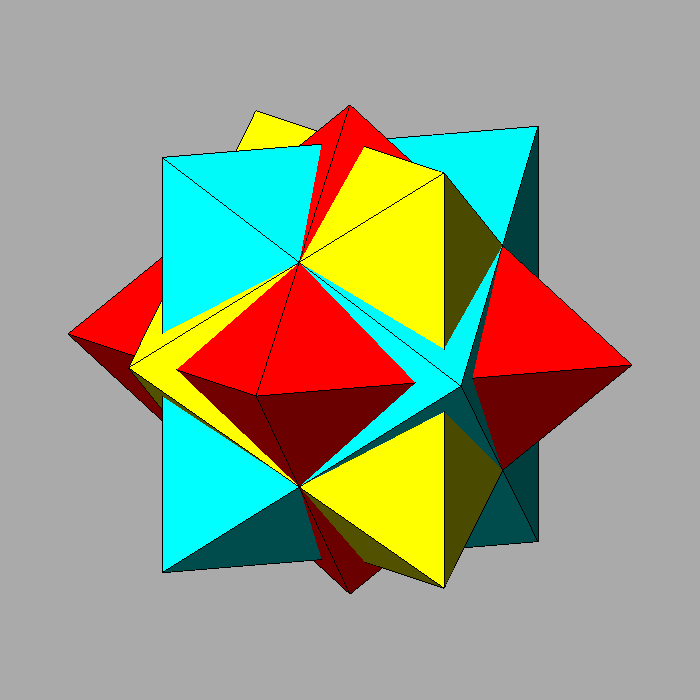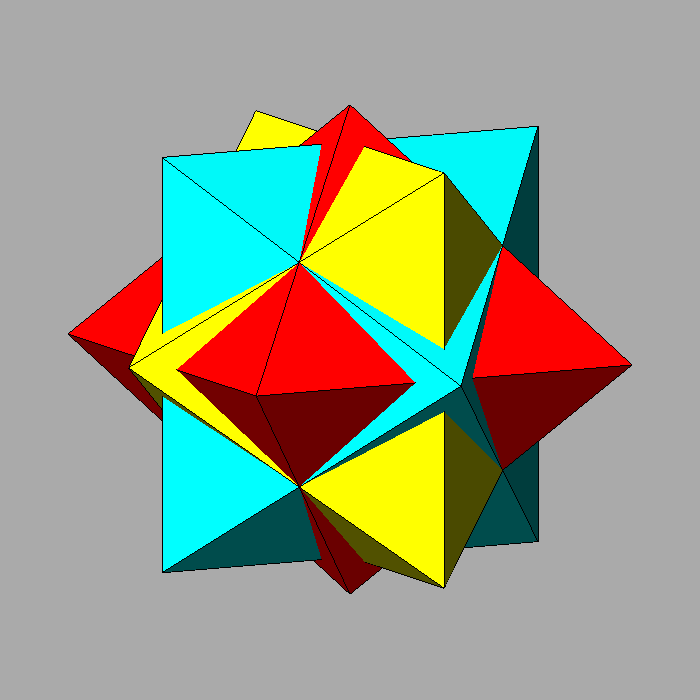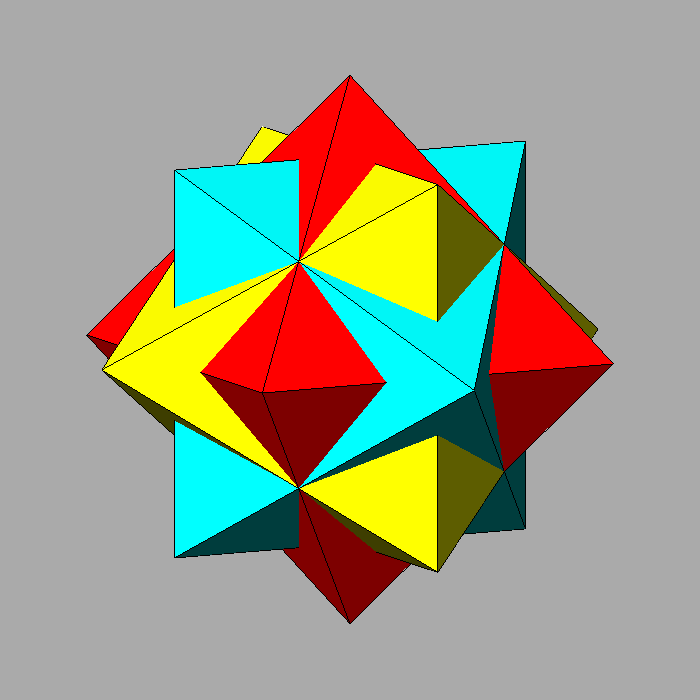
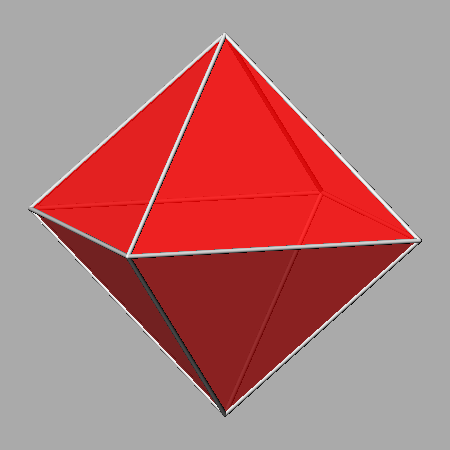
polyhedra constituting it? A possible answer would seem to be: three rotated octahedra
(also taking into account that the compound of three octahedra is the dual of the
compound of three cubes [4,5]).
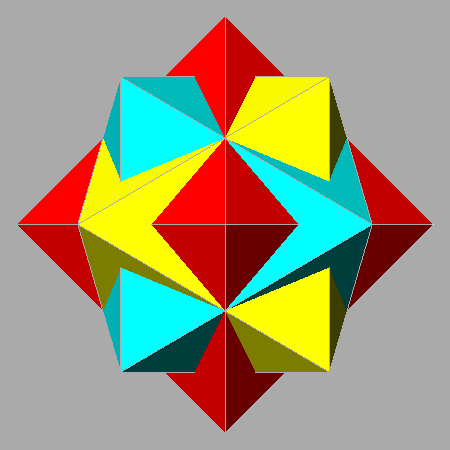
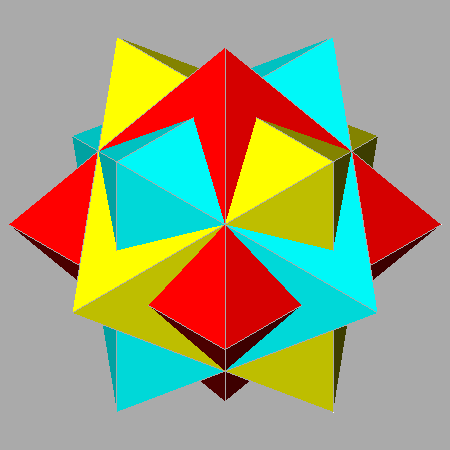
Starting from the red {111} octahedron shown in Fig. 2, a 90° rotation
around the [110] direction leads to the yellow octahedron.
A second 90° rotation of the red octahedron around the [110]
direction leads to the pale-blue octahedron.
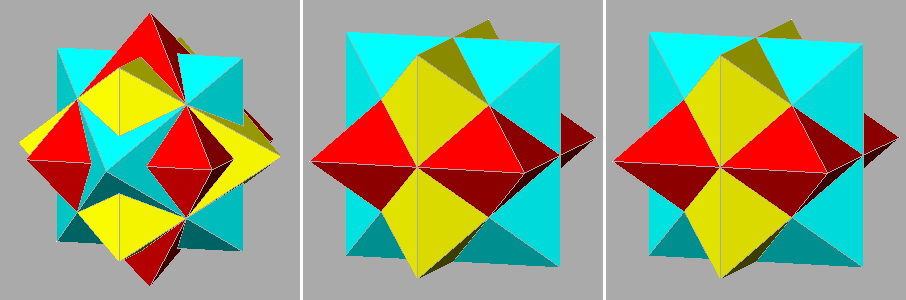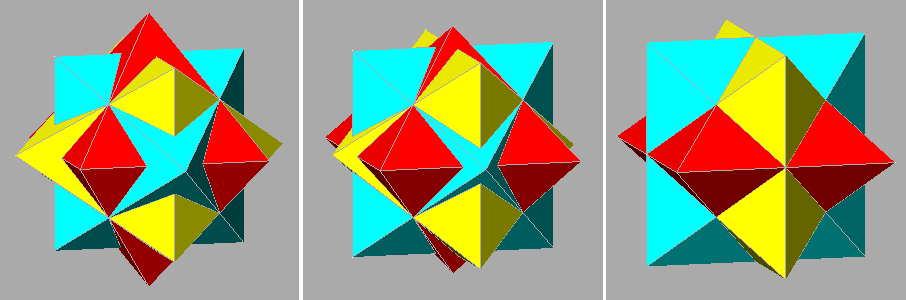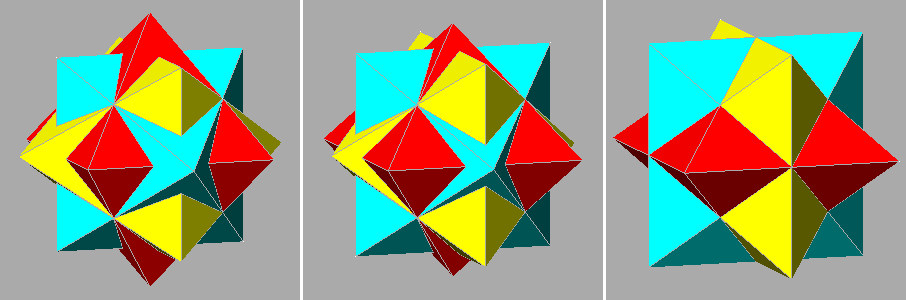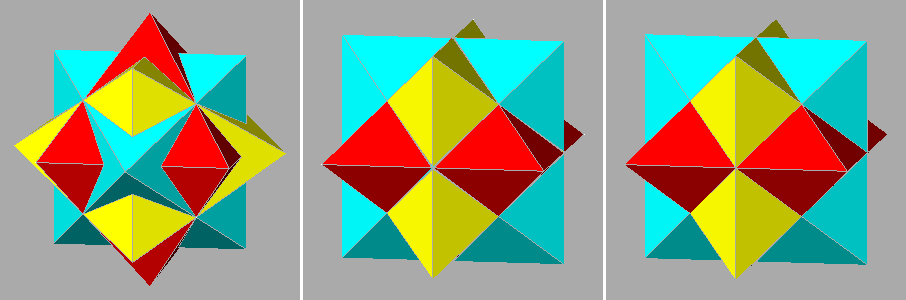
The association of the three octahedra generates a compound polyhedron that
clearly does not coincide with the solid placed on top of the right tower in the Escher drawing.
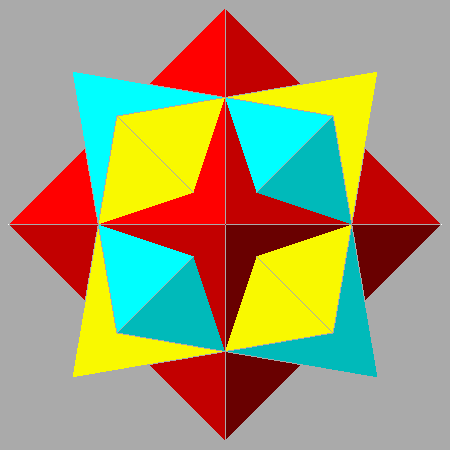
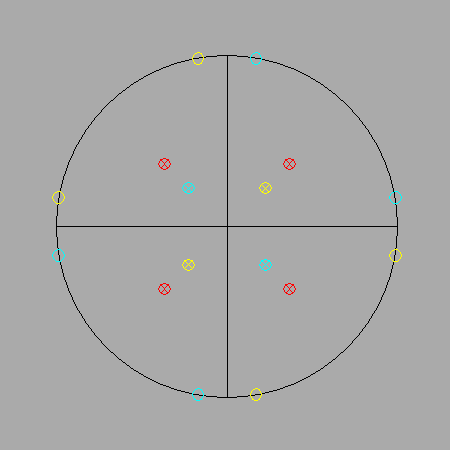
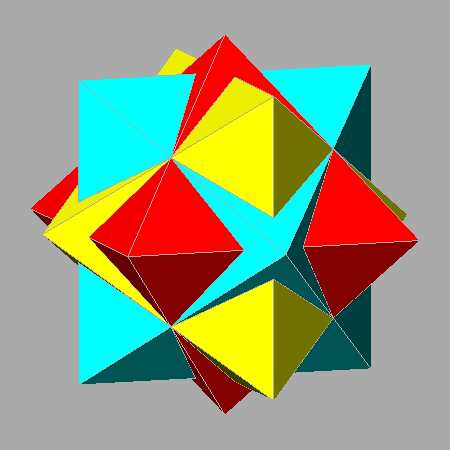
The views of the compound of three octahedra along a 2-fold and a 3-fold axis are reported in the upper row of Fig. 3; the further view along a 4-fold axis and the corresponding stereographic projection of its faces are reported in the lower row.
 |
 |
 |
 |
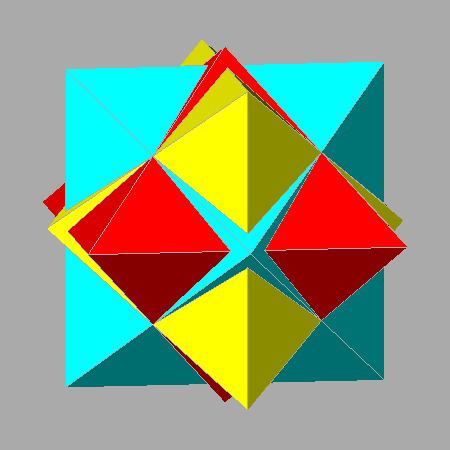
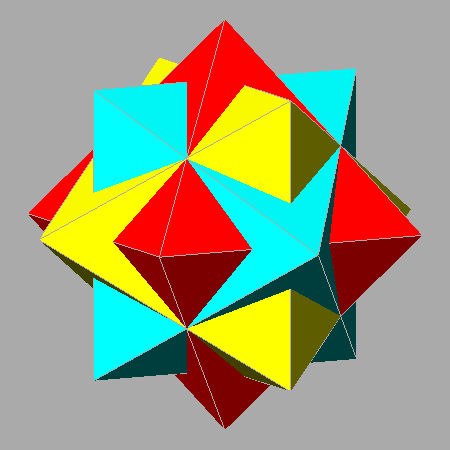
In order to get the Escher's solid, it is necessary to
substitute a flatter square dipyramid for the octahedron.
Then, in Fig. 4,the animated sequence
made of seventeen frames shows a series of compounds of 3 square dipyramids,
obtained decreasing progressively the angle between the normal to the
dipyramidal faces and the vertical [001] 4-fold
axis, from 54.74° = arccos(1/√3),
angle between the normal to the {111} faces of the octahedron and the [001] direction, to the final
value of 45°
= arccos(1/√2).
Considering a monometric system of
Cartesian axes, in correspondence of such 45° angle the Miller's indices relative to the square
dipyramid assume the values {11√2};
the association of this dipyramid with two other square dipyramids, obtained by 90°
rotations around the [110] and [110]
directions, reproduces exactly the Escher solid.
The presence of an irrational value among the Miller's indices is due to the
fact that we are not dealing with a crystal, which would be subject to the
Hauy's law stating that the ratios of the indices are rational numbers.
The faces of the square dipyramid generating the Escher's solid are isosceles
triangles, in which the ratio R between
each of the two equal sides and the third side corresponds to √3
/2 (as one can infer from geometrical considerations relative to Escher's
solid, leading to
 |
The left column of Fig. 5 shows, in succession, the initial {111} octahedron, the final {11√2} square dipyramid and an animated stereographic projection illustrating the transition between the two single forms; in turn, the right column shows the compound of three octahedra, the Escher's solid and an animated stereographic projection illustrating the transition between the two compounds.
It is interesting to note that the skeletal forms of both compounds appear in
different versions of Escher's wood engraving Stars (1948) :
the compound of three dipyramids, together with other polyhedra, in a
preliminary study of the work [6], whereas the compound of three octahedra is
the main subject of the final version [7].
Fig. 6 shows the remake, along the same angle of view, of both skeletal
compounds of three polyhedra drawn by Escher in the successive versions of
Stars.
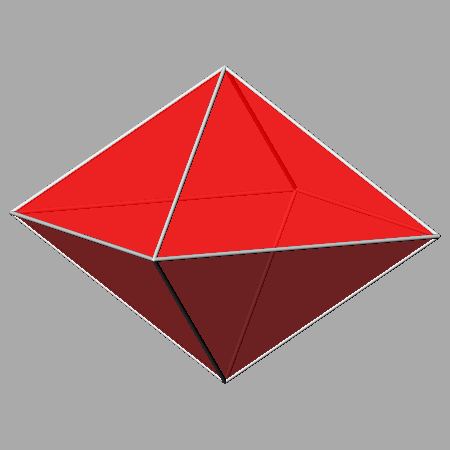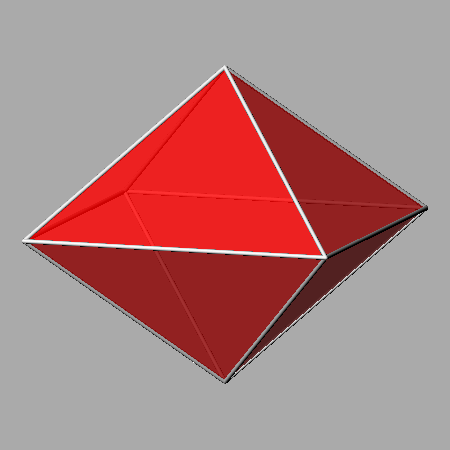
Substantially, the octahedron can be described as a particular square dipyramid whose faces have the shape of equilateral triangles;
because of the presence of four 3-fold axes normal to the faces, in addition to
the three 4-fold axes, the six 2-fold axes, the nine mirrors and the inversion
center [7], the relative point group
symmetry is 4/m 3 2/m.
The faces of each other square dipyramid are instead isosceles triangles: it
implies the absence of the 3-fold axes and therefore the relative symmetry is
lower than the symmetry of the octahedron.
When all the generic {hkl} forms,
neither parallel nor perpendicular to the [001] 4-fold axis, are square dipyramids,
the corresponding point group symmetry
is 4/m.
Applying to the {11√2} square dipyramid
a 45° clockwise rotation around the vertical [001] axis,
one obtains a congruent form characterized by the more usual {101} Miller's
indices: in fact, assuming again a monometric system of Cartesian axes, the
(101) face of the dipyramid intersects equal parameters on [100] and [001] axes.
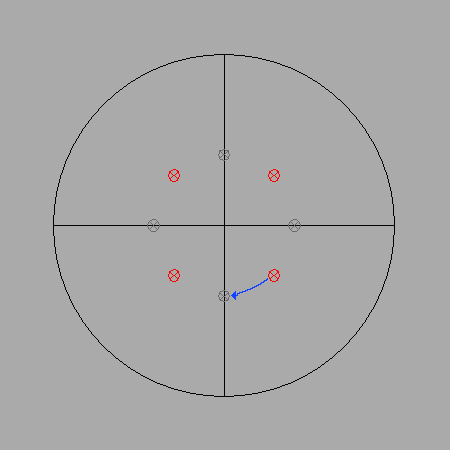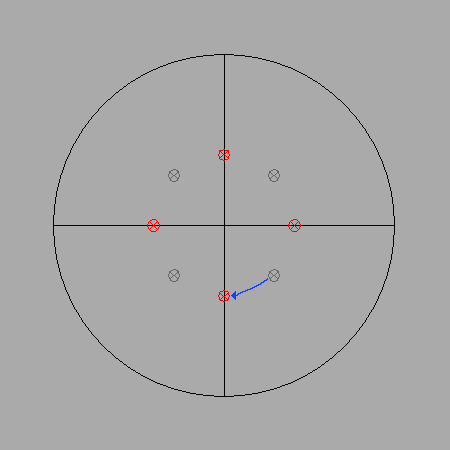
The animated views of the square dipyramids involved in the 45° rotation and
their stereographic representation are shown in Fig. 7 .
 |
 |
Analogously to the procedure previously adopted for the {111} octahedron, a 90° rotation
of the red {101} square dipyramid
around the [100] direction leads to a yellow square dipyramid; another 90° rotation of the red dipyramid around the [010] direction leads to a
The Escher's solid deriving from the square dipyramids in this new orientation
is shown in the fourth image of Fig. 8.
In the upper row of Fig. 9 one can see the three compounds made of two square dipyramids,
intermediates between the single dipyramid and the Escher's solid.
In each case, four couples of faces belonging to different square dipyramids are partially overlapped:
the shared surfaces, differently coloured, have a rhombic shape (with the ratio between the length of the diagonals
equal to √2).
As a consequence, the intersection of the three square dipyramids corresponds to a rhomb-dodecahedron
(viceversa, the stellation of the rhomb-dodecahedron generates the Escher's solid [3]).
In the lower row of Fig. 9 one can
see, on the left, the rhomb-dodecahedron which results from the intersection of
the three square dipyramids generating the Escher's solid drawn on the right.
The central image of the lower row represents the stereographic projection,
along the [001] direction, of the rhomb-dodecahedron and also of the faces of the Escher's solid.
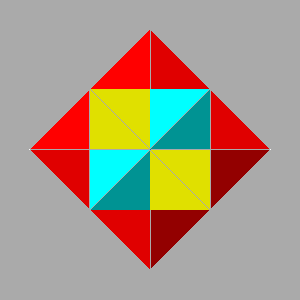
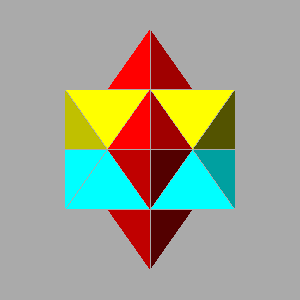
Six further views of the Escher's solid are visualized in Fig. 10: the views along the [001] 4-fold axis, the [111] 3-fold axis and the [110] 2-fold axis (in each case with the [110] direction set vertically) in the upper row, the related clinographic views in the lower row.
 |
 |
 |
_Escher_solid_along_[001]_vert_[1-10]_clino_300px.png) |
_Escher_solid_along_[111]_vert_[1-10]_clino_300px.png) |
_Escher_solid_along_[110]_vert_[1-10]_clino_300px.png) |
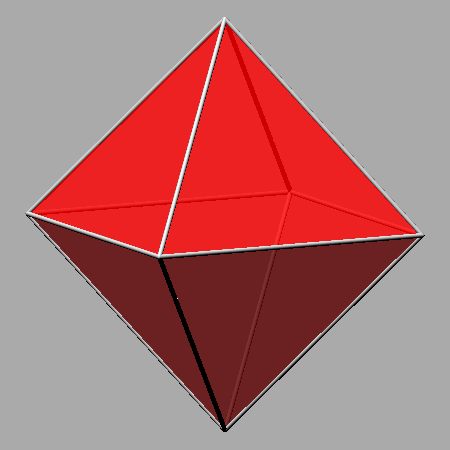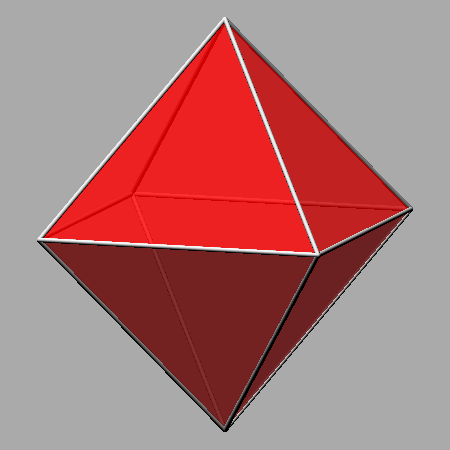
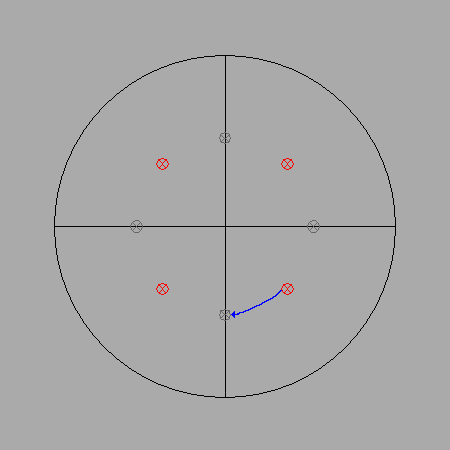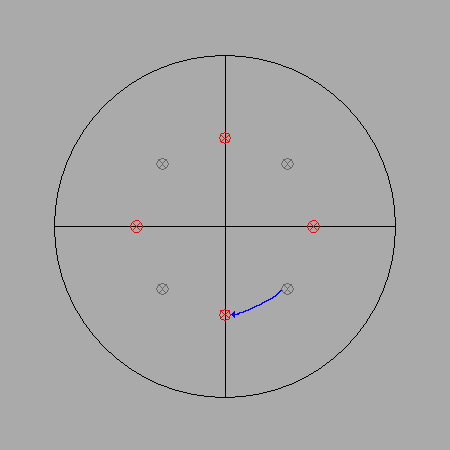
Applying also to the {111} octahedron
a 45° rotation around the vertical [001] axis,
one obtains a congruent form characterized by the irrational {√201}
Miller's indices.
The animated views of the octahedra involved in the 45° rotation and
their stereographic representation are shown in Fig. 11.
 |
 |
The solid derived from the intersection of the three octahedra corresponds to the {√201} tetrakis-cube
(or tetrakis-hexahedron), polyhedron with 24 faces that can be derived from a
cube in which, by a process of cumulation [9]
(or augmentation [10]), a square pyramid
grows on each face of the cube. In Fig. 13
one can see, on the left, the tetrakis-cube, on the right the compound of 3
octahedra of which the tetrakis-cube is the intersection, and, in the center,
the stereographic projection along [001], common to both:
the relative point symmetry is 4/m 3
2/m, higher than the 4/m 2/m
2/m point symmetry of the compound of two octahedra and
of the solid resulting from their intersection.
One can notice that the orientation of all the rotation axes relative to the
compound of three octahedra derived from the initial red {√201}
octahedron is the usual orientation of the rotation axes
in
the 4/m 3
2/m cubic point group, relative also to the tetrakis-cube:
4-fold axis along [100],
On the other hand, in case of the association of three octahedra starting from
the {111} octahedron, as shown previously in the stereographic projection of Fig. 3, the
orientation of the rotation axes looks uncommon: 4-fold axis along
[110], 3-fold axis along [0 √2 1],
In practice, the different orientation
in the two cases of the axes of symmetry consists in a 45° rotation
around the [001] direction.
Incidentally, one must avoid to mistake the previous {√201}
tetrakis-cube for the Catalan {201} tetrakis-cube, dual of the Archimedean
truncated octahedron [11,12], in which the height of the square pyramids augmenting the faces of the
cube is lower than in {√201}
tetrakis-cube.
In Fig.
14, the Catalan tetrakis-cube is drawn in the upper row, on the left:
it corresponds to the intersection of three square dipyramids, whose compound, derived from the
red {201} square dipyramid, is shown on the right.
The dual of the Catalan tetrakis-cube is the Archimedean truncated
octahedron shown in the lower row, on the left, together with the compound of an
octahedron and a cube of which it is the intersection; the ratio between the central
distances of the octahedral and cubic faces is equal to √3/2.
Even if, as already pointed out, the (101) face of the square dipyramid and the (111) face of the octahedron make different angles
with the vertical [001] direction, both belong to the zone of the [101] direction,
like so, in case of the 4/m point group, all the square dipyramids {hkh}
with
The left column of Fig.15 reports a series of six polyhedra from the
{101} square dipyramid to the {111} octahedron, passing through the {hkh}
dipyramids: {414}, {525}, {212}, {434}; the series on the right includes the corresponding compounds of 3
polyhedra, from the Escher's solid to the compound of three octahedra.
 |
 |
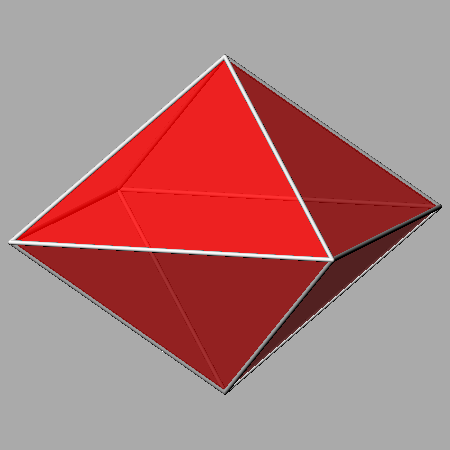
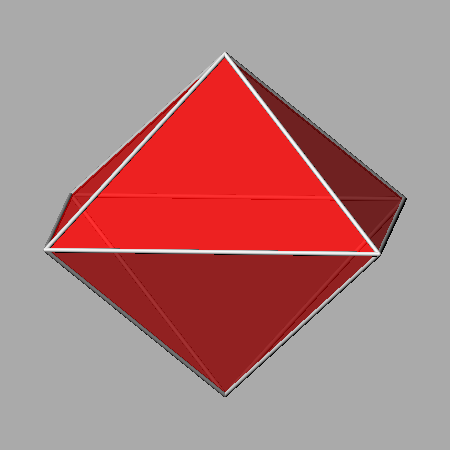
{101} square dipyramid and the relative compound of 3 dipyramids corresponding to
the Escher's solid | |
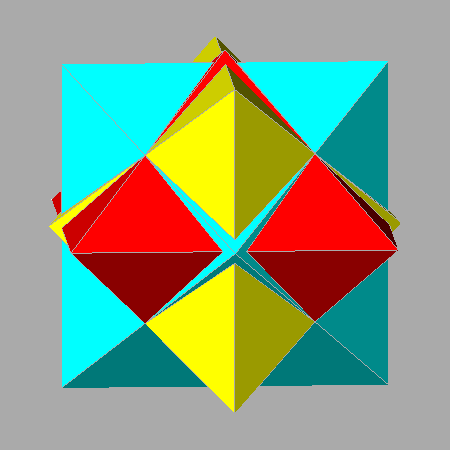
 |
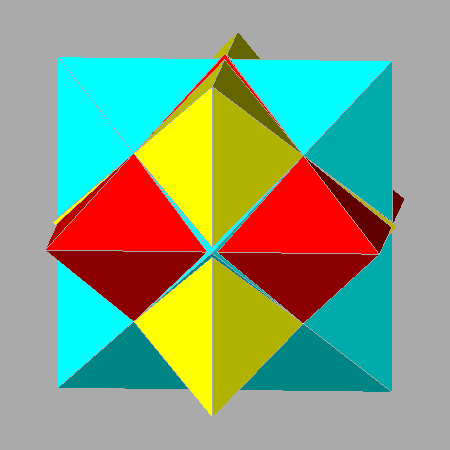 |
{414} square dipyramid and the relative compound of 3 dipyramids | |
 |
 |
{525} square dipyramid and the relative compound of 3 dipyramids | |
 |
 |
{212} square dipyramid and the relative compound of 3 dipyramids | |
 |
 |
{434} square dipyramid and the relative compound of 3 dipyramids | |
 |
 |
{111} octahedron and the relative compound of 3 octahedra | |
The compounds of three dipyramids derived from {hkh} square dipyramids, shown in
Fig.15, are
congruent with the compounds of the sequence reported in Fig.4, relative to compounds of
three dipyramids
derived from {h'h'l'} square dipyramids included between the {111} octahedron and
the {11√2} dipyramid, where: h'/l' = √(k/h)2 +1 /√2 .
It is interesting to remark that if the initial polyhedron is whichever {h0l} square dipyramid, the
two
other square dipyramids belonging to the corresponding compound of three dipyramids can be alternatively obtained by 120° and 240° rotations
of the first dipyramid
around
the [111] direction.
This direction correspond to one of the four 3-fold axes (the others are along: [111],
[111],
[1 1
1] ) present in all the
compounds of three square dipyramids deriving from whichever {h0l} dipyramid, included the Escher's solid derived from the {101} dipyramid, and
in the compound of
three octahedra derived from the {√201}
octahedron.
Similarly, when the initial polyhedron is the {111}
octahedron or whichever {h'h'l'} square dipyramid, included the {11√2}
dipyramid generating the Escher's solid, the 120° and 240° rotations are around the [0 √2 1]
3-fold axis (or one of the three other equivalent directions: [0 √2
1], [√2
0 1], [√2 0
1]) characterizing
these other compounds of three square dipyramids.
Consequently, one can wonder which [uvw] direction corresponds to a 3-fold axis
of each compound of three square dipyramids derived from a square dipyramid
{hkh} with
Relatively to a series of such compounds of three dipyramids, in the four columns of Table 1 one can find, in sequence:
| Rotation axes of the compounds of 3 dipyramids deriving from the {hkh} square dipyramids | |||
| initial square dipyramid {hkh} | 4-fold axis [uv0] | 2-fold axis [u'v'0] | 3-fold axis [u'v'w'] |
| {101} | [100] | [110] | [111] |
| {717} | [710] | [340] | [6 8 5√2] |
| {616} | [610] | [570] | [5 7 √37] |
| {515} | [510] | [230] | [4 6 √26] |
| {414} | [410] | [350] | [3 5 √17] |
| {313} | [310] | [120] | [2 4 √10] |
| {212} | [210] | [130] | [1 3 √5] |
| {323} | [320] | [150] | [1 5 √13] |
| {434} | [430] | [170] | [175] |
| {545} | [540] | [190] | [1 9 √41] |
| {656} | [650] | [1 11 0] | [1 11 √61] |
| {111} | [110] | [010] | [0 √2 1] |
The ratio u/v between the first two indices of the direction [uv0] coinciding with a 4-fold axis of each compound of 3 dipyramids corresponds to the ratio h/k between the {hkl} indices of the dipyramid which generates the compound of three dipyramids: u/v = h/k
The [u'v'0] 2-fold axis of a compound of three dipyramids makes an angle of 45° with the [uv0] 4-fold axis of the
same compound.
It follows that the ratio u'/v' between the first two indices of a direction [u'v'0]
corresponds to the ratio between the difference and the sum of the couples of indices u,v and h,k:
The [u'v'w'] 3-fold axis of whichever compound makes an angle of 54.74° = arccos (1/√3) with the [001] vertical direction (always coinciding with a further 4-fold axis in all the compounds) and a complementary angle of 35.26° = arctan (1/√2) with the horizontal [u'v'0] 2-fold axis of the same compound of 3 dipyramids. From:
and therefore, taking into account the ratio u'/v' previously calculated,
the values of the [u'v'w'] indices relative to one of the four
The directions of the three other 3-fold axes of the compound of 3
dipyramids can be obtained by sequential 90° rotations of the first
As already pointed out for the {h0l} and {hhl} square dipyramids, all the compounds
of three dipyramids which derive from {hkl} square dipyramids, whose indices are characterized by the
same h/k ratio, share all the directions of the rotation axes; for istance, in the compounds
of three dipyramids deriving from initial {43l} square dipyramids,
that is with h/k = 4/3 and variable values of l, a 4-fold axis is directed along [430], a 2-fold axis
along [170] and a 3-fold axis along [175].
In Table 2 one can see the complete list of the rotation axes relative to the compounds of three dipyramids
derived from {hkl} square dipyramids in which
|
Complete list of the rotation axes present in the compounds of 3 dipyramids deriving from
an initial {hkl} square dipyramid in which h/k = 4/3 | ||
| three 4-fold axis | six 2-fold axis | four 3-fold axis |
| [001] , [430] , [340] | [170] , [710] | [175] , [715] , [1 7 5] , [715] |
| [435] , [345] , [4 3 5] , [345] | ||
Since each of the last four 2-fold axes in the list makes an angle of 45° with the couples
of 4-fold axes [001] and [430] or [001] and [340],
the indices of the corresponding direction can be identified unambiguously.
The left image in the upper row of Fig.16 visualizes an animated sequence which includes a series of compounds of
three square dipyramids,
each deriving from a {hkl} square dipyramid characterized by the ratios:
h/k = 4/3 and 1/12
≤ l/k ≤ 16 ;
it was necessary to rescale progressively the frames of the sequence when l/k
≤ 1 because the dipyramids were becoming always more sharpened.
The other images in the figure consist in three frames of the sequence which are
particularly noteworthy: the
right image of the upper row shows the compound generated from the {434}
square dipyramid, belonging to the zone of [101]
direction and intermediate between the compound generated from the
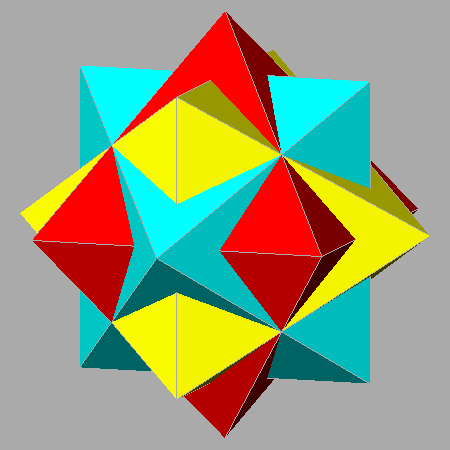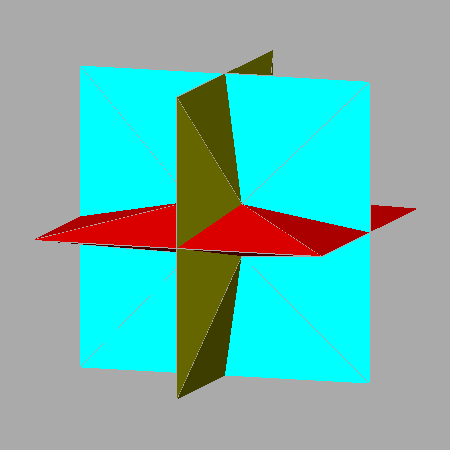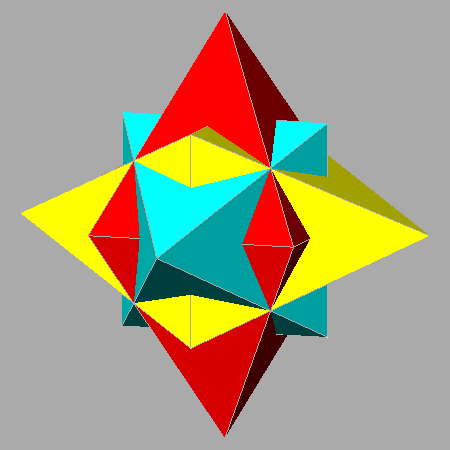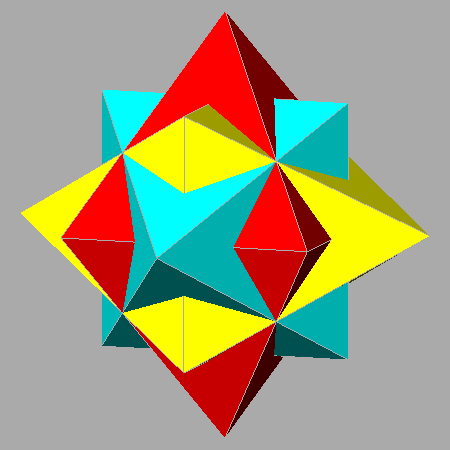 |
_compound_3square_dipyramids_434.png) |
_compound_3octahedra_8.6.5sqrt2.png) |
_compound_3square_dipyramids_435_Escher's_solid.png) |
More
in general, concerning
the series of {hkh} square dipyramids with
Since the normal to their faces makes angles of 54.74° and 45°, respectively,
with the vertical [001] 4-fold axis, from:
|
{h k
√h2
+ k2)/2} initial octahedra
belonging to each compound of 3 octahedra |
{hkh} polyhedra in
zone with [101] having {hkh} indices such as: 0 ≤ k/h ≤ 1 |
{h k
√h2
+ k2} initial square dipyramids belonging to each compound of 3 dipyramids |
| {√2 0 1} | {101} | {101} |
| {715} | {717} | {7 1 5√2} |
| {12 2 √74} | {616} | {6 1 √37} |
| {5 1√13} | {515} | {5 1 √26} |
| {8 2 √34} | {414} | {4 1 √17} |
| {3 1 √5} | {313} | {3 1 √10} |
| {10 4 √58} | {525} | {5 2 √29} |
| {4 2 √10} | {212} | {2 1 √5} |
| {6 4 √26} | {323} | {3 2 √13} |
| {8 6 5√2} | {434} | {435} |
| {10 8 √82} | {545} | {5 4 √41} |
| {12 10 √122} | {656} | {6 5 √61} |
| {111} | {111} | {1 1 √2} |
In Fig.17, the left and the right images consist in the animated sequences
of frames describing the 45° rotation underwent by the compound of three
octahedra and the Escher's solid, respectively.
In the central image, the first frame of the sequence concerns the compound of 3
dipyramids (derived from the {101} square dipyramid) corresponding to an
Escher's solid, that coincide with the first frame of the third sequence, whereas the last
central frame
concerns the compound of 3 octahedra (derived from the {111} octahedron) that
coincides with the the last frame of the first sequence.
 |
Links and references
2) www.petergh.f2s.com/escher.html
3) mathworld.wolfram.com/EschersSolid.html
4) mathworld.wolfram.com/Octahedron3-Compound
5) www.georgehart.com/compound-cubes-index.html
6) J. L. Locher, Le monde de Escher ( pag.151), Chêne (1971)
7) www.georgehart.com/escher.html
8) International Union of Crystallography
International Tables for Crystallography, Vol. A, Theo Hahn
Editor, Kluwer Academic Publisher (1989)
9) mathworld.wolfram.com/Cumulation.html
10) Peter R.Cromwell, Polyhedra (pag. 195), Cambridge University Press, New York (1997)
11) www.georgehart.com/archimedean-duals-info.html
12) L.Zefiro and M.R. Ardigo', Platonic and Catalan polyhedra as archetypes of forms belonging to the cubic and icosahedral systems, Visual Mathematics, Vol. 11, No. 2, 2009