|
INTERSECTIONS OF ART AND SCIENCE IN ANCIENT AND MODERN
GRAPHICS
SZ. BÉRCZI and S. KABAI
Name: Szaniszlo Bérczi (b. Szeged, Hungary, 1950) Address: Eötvös University, Dept. G. Technology, Cosmic Materials Space Research Group, H-1117 Budapest, Pázmány Péter sétány 1/a, Hungary E-mail: bercziszani@ludens.elte.hu Fields of interest: Geometry, ethnomathematics, ornamental arts, planetary science, materials science. Publications and/or Exhibitions: Bérczi Sz. (1989) Symmetry and technology in ornamental art of old Hungarians and Avar-Onogurians from the archaeological finds of the Carpathian Basin, seventh to tenth century A.D., In: Symmetry 2, Ed .I. Hargittai, Oxford: Pergamon Press, and Computers Math. Applic. (CAMWA), 17, No. 4-6, 715-730. Bérczi Sz. (1993) Symmetry and topology in cellular automatic transformations: The solution of the indirect von Neumann problem for the transfigurations of cylindrical cell mosaic systems of Fibonacci plants, Abstracta Botanica (Budapest), 17 (12.). Bérczi Sz. (1993) Symmetry changes by cellular automata in transformations of closed double-threads and cellular tubes with Möbius-band, torus, tube-knot and Klein-bottle topologies, Symmetry: Culture and Science, 4, No. 1, 49-68. Bérczi Sz. (2000) Katachi U Symmetry in the ornamental art of the last thousands years of Eurasia, Forma (Tokyo), 15/1, 11-28. Bérczi Sz. (2000) Double layered equation of
motion, HyperSpace, 9, No. 3, 45-63.
Name of coauthor: Sandor Kabai (b. Báránd, Hungary, 1950) Address: UNICONSTANT, H-4150 Püspökladány, Honvéd u. 3. Hungary E-mail: unico@mail.matav.hu Fields of interest: Geometry, ornamental arts, mathematics. Publications and/or Exhibitions: Kabai S. (1999): Math Art, [Exhibition], Püspökladány, Hungary, September 1999. Bérczi, Sz., Kabai, S., and Pataki, T. (2000) Role of katachi in developments of abstract design, Forma (Tokyo), 15/2,181-186. Bérczi Sz. and Kabai S. (2000) From Eurasian Communal Art to Computer Graphics, Vámospércs, Hungary: Piremon Press. Bérczi Sz., Kabai S., Karsai J., Gévay G., Pataki T., and Szilassi L. (2001) Reale und virtuelle Welten, [Exhibition], Berlin: University of Applied Sciences, April 3-5, 2001. Bérczi Sz., Kabai S., Karsai J., Gévay
G., Pataki T., Szilassi L., Pályi B. (2001): Reális és
virtuális világok, [Real and Virtual Worlds, in Hungarian;
Exhibition], Szeged, Hungary: University of Szeged, April 21-May 4, 2001.
Abstract: Phenomena of nature trigger communities
to draw natural patterns. These prints about nature reflect knowledge of
the community in that period. Selecting "eternal" interesting objects we
may follow rebirth of natural forms or phenomena in new community arts
till today. In our paper artifacts are compared: those designed in ancient
communal arts with those made in modern computer graphics. We focus on
architecture.
1. OLD THINKING, OLD ART AND THE SKY Imagination of ancient people reconstructed the world in myths. Events on the earth were in mythical correspondence with calendar events, mostly visible in the sky. But even mythic thinking used conservation principles. Stable frequent phenomena played the basic role in forming the thread of invariance in the related mythic stories. Even if the recognitions were through analogies, symbols and legends, the core of this stories were real nodes of life (birth, marriage, family, death). All these events were projected on a parallel thread of
motions on the sky. The of the evening sky with stars, Moon, planets, was
the most attractive phenomena. Ancient art expressed this mythic correspondence
between terrestrial and celestial life and art was stimulated to find more
and more symbolic representation of these relations. Through the Tree of
Life we can reach heavens. Birds fly to carry our souls to the heavens.
Even the church towers of medieval ages spire toward the sky. Church gates
expressed celestial order along their arcs in their tympanons. Sometimes
even the eclipses were referred by dragons "eating" the Sun or Moon at
the nodes of their celestial pathways. Sequence of scenarios in the fresco
story on the walls preserved mythic fight between light and darkness (Legend
of St. Ladislaus in Hungary). Flowers in the boarded ceilings of old churches
in the Renaissance architecture symbolized stars and heavenly order and
beauty. 1000 years ago we could read our life-story according to the events
among the constellations. Folk tales were also organized according to this
mythic line of thinking (Bérczi, Kabai, and Pataki,
1999).
2 ARCHITECTURE AND COMPUTER GRAPHICS (Graphics of Sandor Kabai) Computer world helps us in the twenty-first century to
prefer complex understanding of phenomena of nature. With modern computer
graphics we can imagine and construct new worlds. This capability comes
from understanding the basic constructional relations and rules. To draw
real structures we operate mathematics, which describe objects by formulated
steps. Real, visible, enjoyable, true objects are also the aim of the modern
graphical representation. That is science which extremely appreciates the
valid representations, but added beauty rise the worth of graphical appearance
of the constructed objects. (Takaki et al., 1996). These
all are involved in Mathematica, which was used by Sándor Kabai
in making his Math-Art.
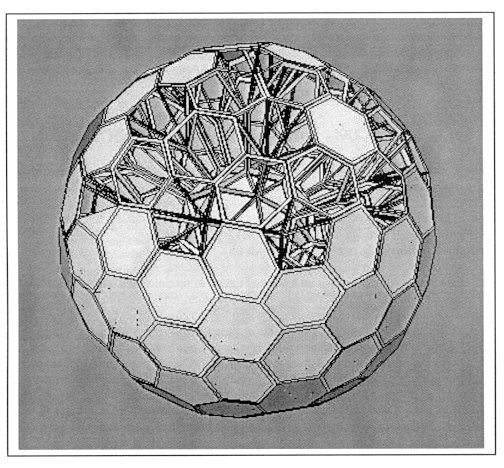
Fig. 1. Space Station: computer graphics of Sándor Kabai. We feel that we can understand more about the world, in
constructed objects from computer graphics? The visible objects are only
small number of the representatives of a great realm of similar ones. Computer
graphics can animate the imagined variants, can help in visualising the
world of imagination! We can see computer constructed space stations floating
in space. Sunlight illuminates them, shades emphasize their spatial order
(Bérczi and Kabai, 2000).
REFERENCES Bérczi Sz. and Kabai S. (2000) From Eurasian Communal Art to Computer Graphics, Vámospércs, Hungary: Piremon Press. Bérczi Sz., Kabai S., and Pataki T. (1999) Role of katachi in development of abstract design, Exhibition and Lecture at KUS 2 Symposium, Tsukuba, Japan, November 17. Takaki R., Arai M., and Utumi M. (1996)
How to promote the morphological sciences, In: Katachi U Symmetry,
(T. Ogawa, K. Miura, T. Masunari, D. Nagy, Eds.), Tokyo: Springer, 143-154.
|