DECORATIONS
BY MEANS OF GENERALIZED CLOTHOIDS
(Italy)
Abstract
1. Introduction
The clothoid, also known as Cornu spiral or Euler spiral, is
a plane curve which is often applied in optics, for diffraction studies, and in
the design of civil constructions, like roads and railway lines, when it is
necessary to create a connection between a straight stretch and a bend. Its
characteristics and main properties are described in many textbooks or
web-sites (see for example [1], [2]). Its equation,
expressed in cartesian parametric form by means of the Fresnel integrals, is:
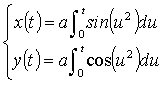 (1)
(1)

The property of having the
curvature proportional to the arc lengthis the main characteristic of the clothoid.
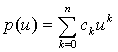 (2)
(2)
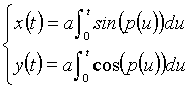 (3)
(3)
Figs.2a, 2b, 2c show some examples of curves that can be plotted
starting from equations (3). The characteristic of these curves of being at the
same time curly and symmetrical makes them suitable to design some decorations that
we are going to present in the next paragraph.
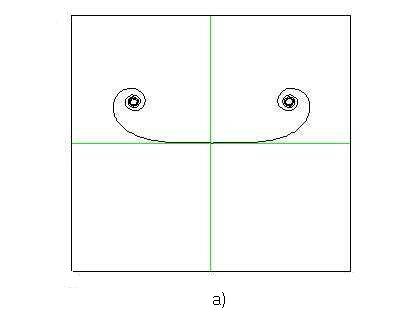
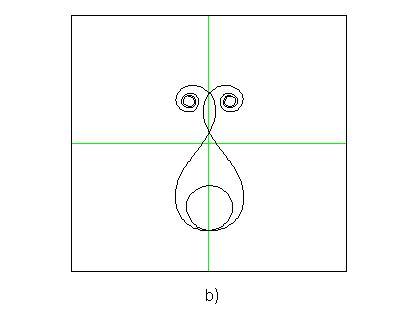
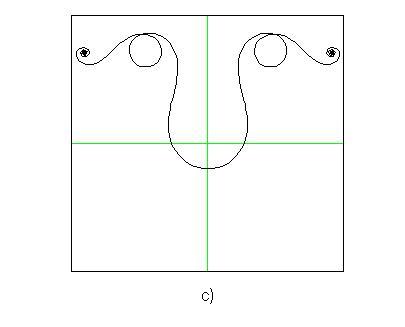
It is interesting to note that curves similar to the ones shown in Fig.2
can quite often be found as decorations in metal gates, barred windows and
fences.
It is worthwhile to mention that also different functions from polynomials
can be used to obtain generalized clothoids;
thus, being f(u) a continuous function together with
its first derivative, we can write:
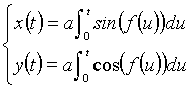 (4)
(4)
Related to this point, we just would like to mention that the artist J.
P. Hébert, created some art-works by drawing in
the sand, by means of a special installation, some curves that can be
considered generalized clothoids according to the equations
(4). (See [3] for more
details).
Finally, it can be shown that the curvature k(u) of the generalized clothoid
is related to the function f(u) by means of the following relation:
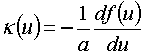 (5)
(5)
In the following we shall consider, according to equations (3), only
polynomials.
3. Creating decorations
Three are the steps which can be followed in order to create decorative
figures starting from generalized clothoids:
generate any number N-1 of daughter curves by
applying to the mother curve the typical geometrical transformations like
rotation, reflection, homothety;
plot together mother and daughter curves in order
to obtain the decoration.
Clearly the choice of the mother curve and of the number of daughter
curves, as well as their disposition, is up to the personal aesthetic taste in looking at
the final result obtained.
4. Examples

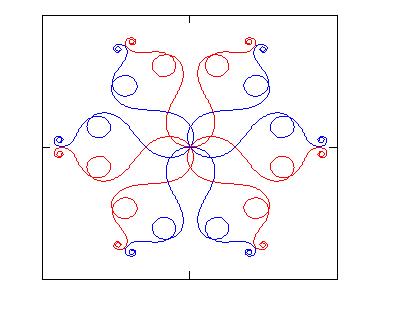
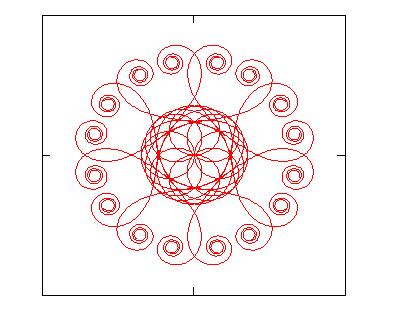
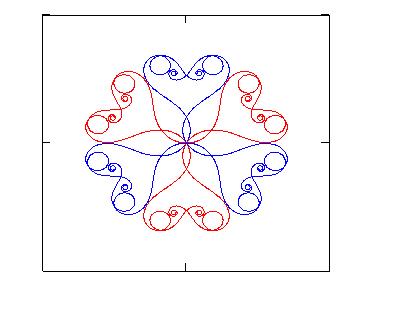
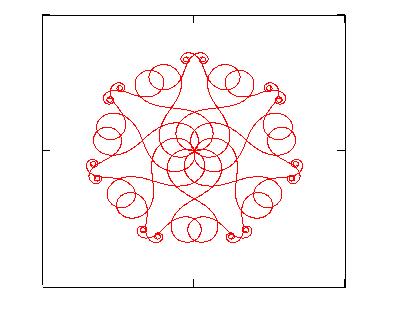

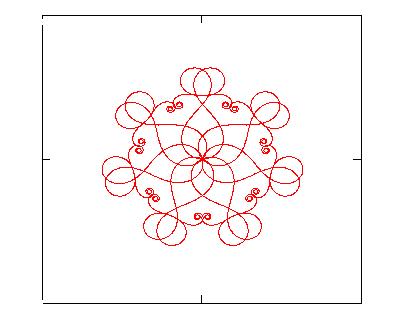

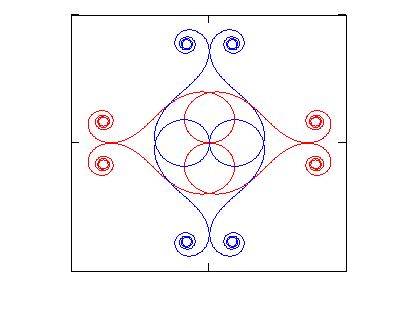
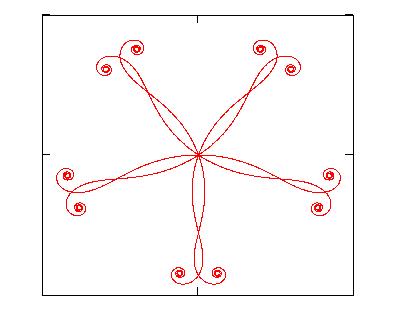
|
Fig. |
p(u) |
N |
[tmin, tmax] |
|
3 |
u5-6.1u3+3.1u |
4 |
[-2.262,
2.262] |
|
4 |
u5-6.u3+5.4u |
6 |
[-2.356,
2.356] |
|
5 |
0.333u3-4u |
8 |
[-4.712,
4.712] |
|
6 |
u5-6u3+4.32u |
7 |
[-2.356,
2.356] |
|
7 |
u5-6u3+7u |
7 |
[-2.356,
2.356] |
|
8 |
2.4u5-6u3+7u |
7 |
[-1.728,
1.728] |
|
9 |
2.4u5-9.3u3+6.7u |
7 |
[-1.948,
1.948] |
|
10 |
2u5-10.5u3+4u2 |
7 |
[-1.005,
2.042] |
|
11 |
0.333u3-3.275u |
4 |
[-4.712,
4.712] |
|
12 |
u5-u |
5 |
[-1.885,
1.885] |
Table I: Data used for generating the figures shown in the paper.
References
[2]http://mathworld.wolfram.com/CornuSpiral.html
[3]http://www.mathematica-journal.com/issue/v7i3/graphics/