|
Subdivision of Space By Ami Korren
Name: Ami Korren, Architect, (b. Tiberias, Israel, 1950). Address: Faculty of Architecture & Town Planning, Technion. Israel Institute of Technology, Technion City, Haifa 32000, Israel. E-mail: Korren-a@inter.net.il Fields of interest:Architecture,
Morphology, geometry, architectural crystallography, and Minimal and consequential
forms.
Publication and exhibition: A.Korren, Periodic 2-manifold surfaces that divide the space into two identical subspaces, Master thesis, Technion, Israel, 1993. M.Burt & A.Korren,Periodic Hyperbolic Surfaces and Subdivision of 3-Space, Katachi U Symmetry, Springer-Verlag, Tokyo, 1996. M.Burt & A.Korren, Self-Dual Space Lattices, and Periodic Hyperbolic Surfaces, Symmetry Natural and Artificial, Symmetrion Budapest, 1995. Science in the Arts
Art in the Sciences, Ernst Museum, Budapest, 1999.
Introduction The morphological dealing with space subdivision by continuous 2-manifold surfaces is one of the important issues for understanding organized space and its order. The phenomenon of periodic surfaces that divide the space into two identical subspaces was dealt with over the years by various researchers from different scientific fields, such as mathematicians, architects, crystallographers, physicians, etc. These periodic 2-manifold surfaces are continuous and divide the space into two identical subspaces, which are graphically characterized by two dual tunneled space networks. These surfaces have the shape of a sponge structure. All the known surfaces were discovered
in an empiric way by various scientific researchers. All in all there were
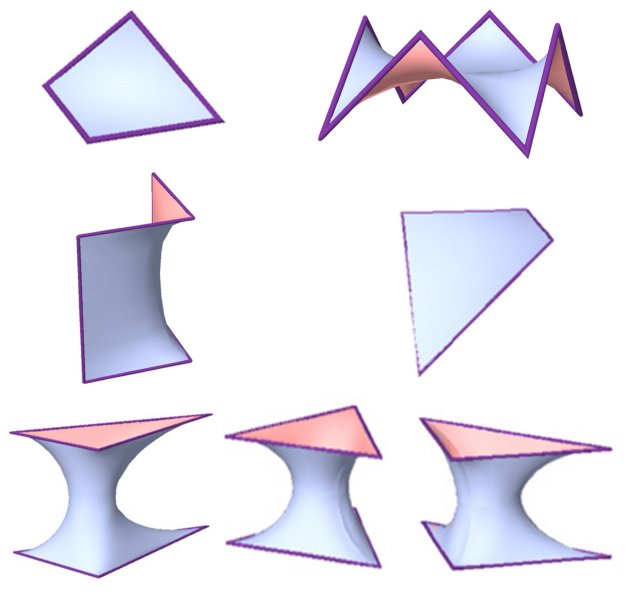
seven topologically different surfaces. (Figure 1)
Figure 1 The seven topologically different surfaces
The goal of the research of the aforementioned phenomenon was to identify the 2-manifold, classify and exhaust them. A systematic method was suggested. The method was strongly based on the theory of symmetry groups. It was assumed that the number of surfaces, topologically different, is finite because of the fact that the number of symmetry groups is finite. Using the method for the exhaustive search the seven known topologically different surfaces were discovered. Only this time, it was done in a systematic way. Topologically different means that the two dual tunnel networks have different shapes, which are the identification mark of the surface. This identification of the 2-manifolds which divide the space into two identical subspaces point out that there is a direct correspondence between the continuous partition surface, the character of the two complimentary subspaces and the two self dual lattices representing them. This phenomenon of the two identical subspaces,
the surface in between, and the two self-dual lattices representing them
makes the exhaustive search of any of them as one and the same problem.
While studying this phenomenon the idea to search for the self-dual lattice
pairs first arose for reasons that will be mentioned later. This approach
led the author to discover a new unknown self-dual space network and a
new 2-manifold surface in between. This new surface has the same characterization
as the seven known surfaces although those seven are still unique. All
the seven surfaces are the product of a simulated dipping process of a
closed wire perimeter (the elementary periodic unit) in a soap solution,
for producing a smooth non-self intersecting hyperbolic minimal surface.
(Figure 2)
Figure 2 The seven elementary periodic units.
This paper intends to report on a research
in which generation of dual space lattices and hyperbolic partition surfaces
are at the core of the inquiry. This research is still in its initial stages.
Currently, the impression is that the number of the dual and identical
lattice pairs and the associated periodic hyperbolic surfaces, partitions
that subdivide the entire space into two complementary and identical subspaces,
is infinite.
The phenomena of 2-manifolds surfaces which divide the
space into two idenical subspaces
Researching the pheonomena of periodic
2-manifolds that lie between two identical-dual subspaces led us to finding
the main charasteristics of these surfaces:
We have chosen to discuss 2-manifold
with 2-fold (1800) rotation symmetry axes. These axes generate
a space lattice, which should be called "the 2-fold axes lattice". This
lattice is periodic, and as such has all the periodicity generating properties,
meaning a 3-dimensional repetitive cell unit and elementary periodic region
(E.P.R.).
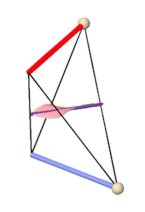
The E.P.R.(Elementary Periodic Region) approach The E.P.R. is the smallest space unit (fundamental region) derived from the Euclidean space by means of the symmetry group that acts on this space. The E.P.R. contains a complete represantion of all the phenomena taking place within the "periodic complex" and particularly, representaion of the whole periodic space, its symmetry group, the 2-fold axes network, the two complementary (identical) subspaces, the self dual lattices-pair characterizing them, as well as the partition surface in between. Identification and exhaustive enumeration
of the E.P.R.s are based on the fact that the number of the symmetry groups
is finite (230 symmetry groups).
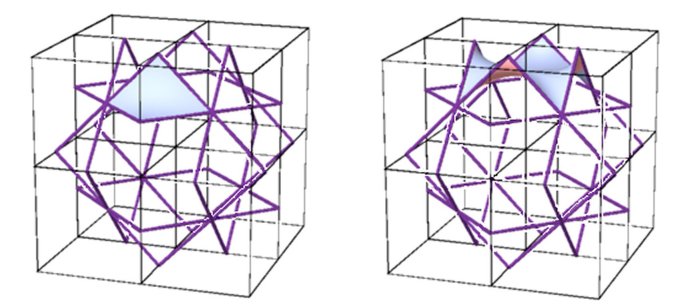
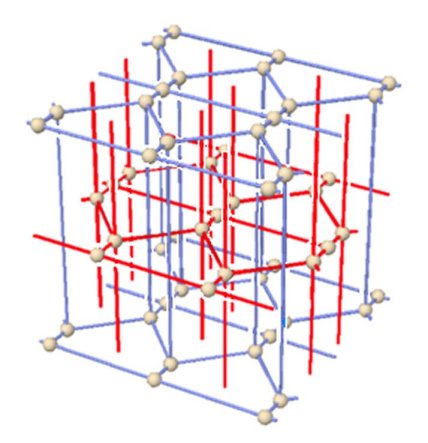
Figure 3 Elementary Periodic Region
The exhaustive method The method of exhaustive search develops in steps as follows:
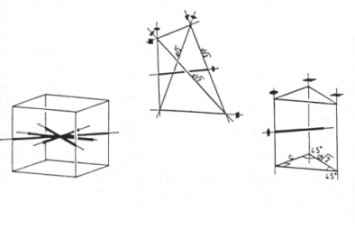
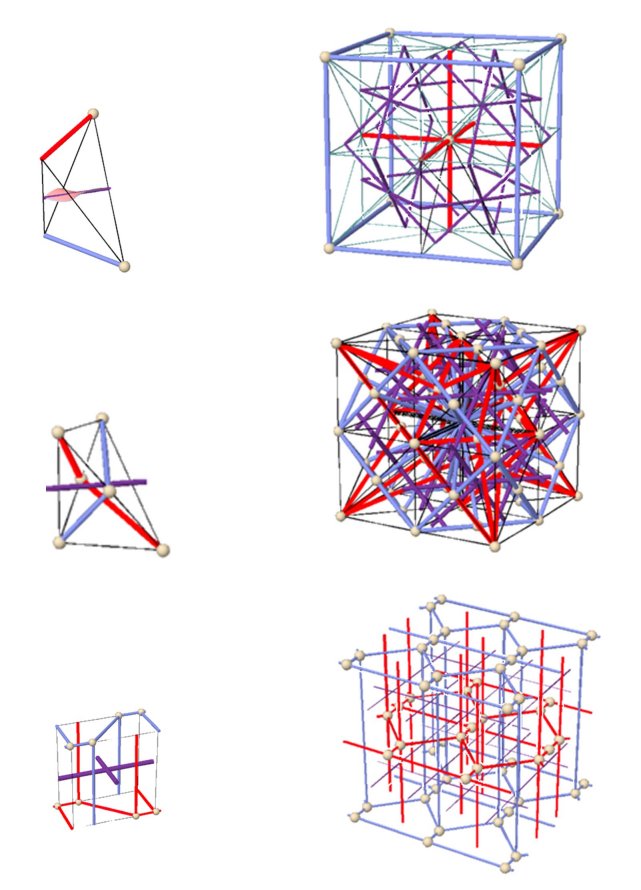
Figure 4 Typical E.P.R.s.
Figure 5 Different E.P.R.s with 2-fold axes
Figure 6 2-fold lattice
Figure 7 Fundamental
closed boundary perimeter(s)
Figure 8 Part of the
2-manifold, which results from a repetitive
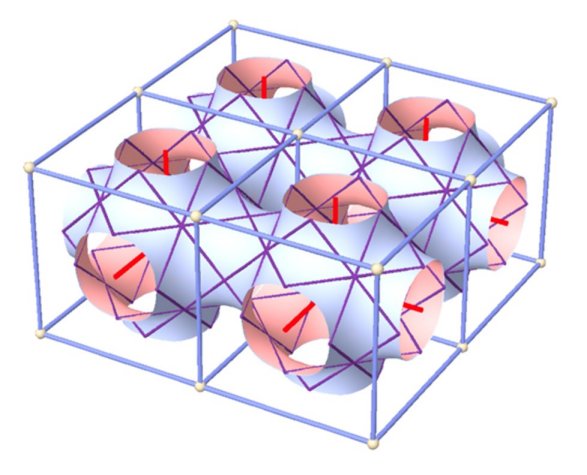
Figure 9 - Identification
of the two dual networks,
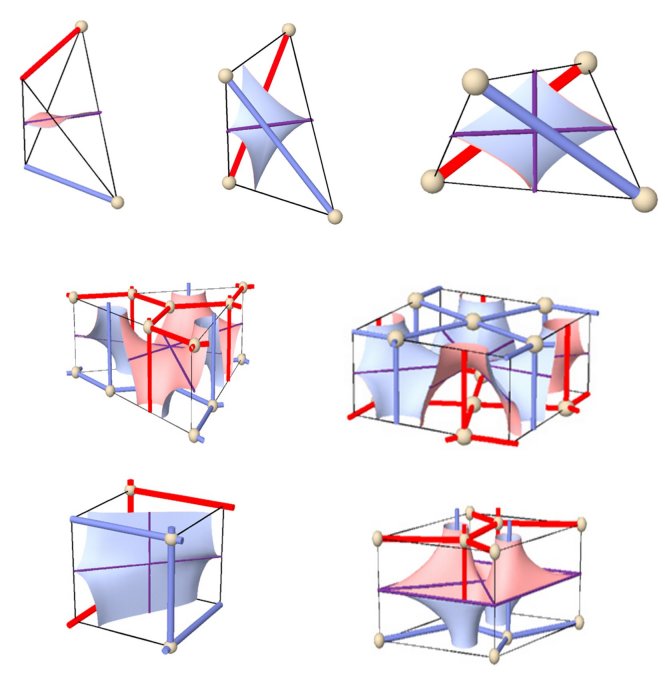
Figure 10 An E.P.R. that represents all periodic elements.
Classification of the 2-manifolds The aforementioned method of searching for the 2-manifolds which divide the space into two identical subspaces, lead to the discovery of many 2-manifolds, with some being topologically similar, and it also lead to exhausting all the 2-manifolds that are topologically different. There are two physical characteristics
to the 2-manifolds which divide the space into two identical subspaces.
One is the form of the translation cell of the 2-manifold. The other one
is the geometric form of the two complementary dual subspaces, which are
represented by the two dual networks.
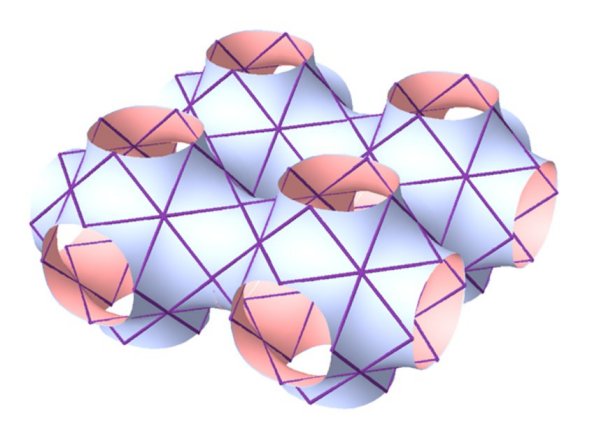
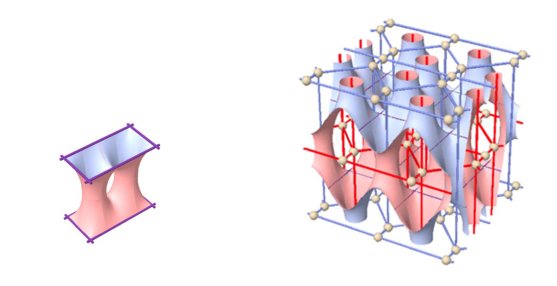
Figure 11 - a.
Two identical dual networks, which
b. Typical translation
cell of a periodic surface that
The two complementary dual subspaces have a maze-like form of two continuous tunnels. The axes of the tunnels form a continuous periodic network, which is called the "tunnel network". The relation between the two networks is complementary (dual) and each one can be defined through the other. The two aforementioned characteristics are derived from one another. The duplication of the transformation cell will lead to the creation of the 2-manifold, which divides the space to two identical subspaces, vice-versa. There is a direct, one to one correspondence between the typical translation cell and the tunnel networks. 2-manifolds, which might seem different from one another, and their fundamental region, are represented within different E.P.R.s. Similar tunnel networks may characterize them, in which case they would lead to a similar typical translation cell. 2-manifolds that are characterized by similar tunnel networks are topologically similar. Classifying the 2-manifolds according to the tunnel networks or the typical translation cell leads to discovering the 2-manifolds that are topologically different from one another. Using the aforementioned searching and
classifying method lead to finding seven topologically different 2-manifolds.
Figure 12 The seven
two identical dual tunnel
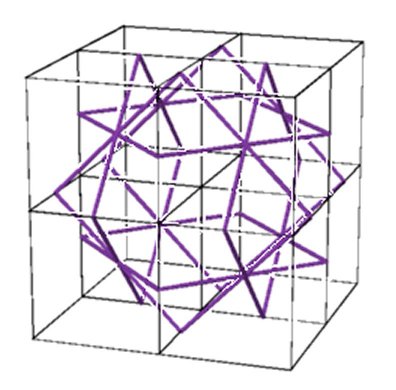
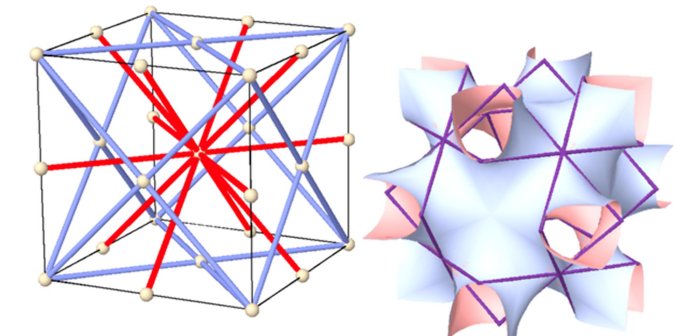
The fact that the tunnel networks characterize the 2-manifolds on a direct, one to one correspondence leads to the conclusion that finding two self-dual networks means finding the surface partition in between. Using empiric search for dual networks
we have found a new unknown pair of self-dual networks, which are topologically
different from the other seven that were found by the aforementioned search
method.
Figure 13 New self-dual networks.
The 2-manifold in between the two new
self-dual networks is also topologically different from the other seven
2-manifolds. The fundamental 2-manifolds that are bounded by the 2-fold
closed boundary perimeter are also unique. While the first seven 2-manifolds
could be a product of a simulated dipping of a closed perimeter in a soap
solution, the new one cannot.
Figure 14 New 2-manifold
in between two self-dual networks
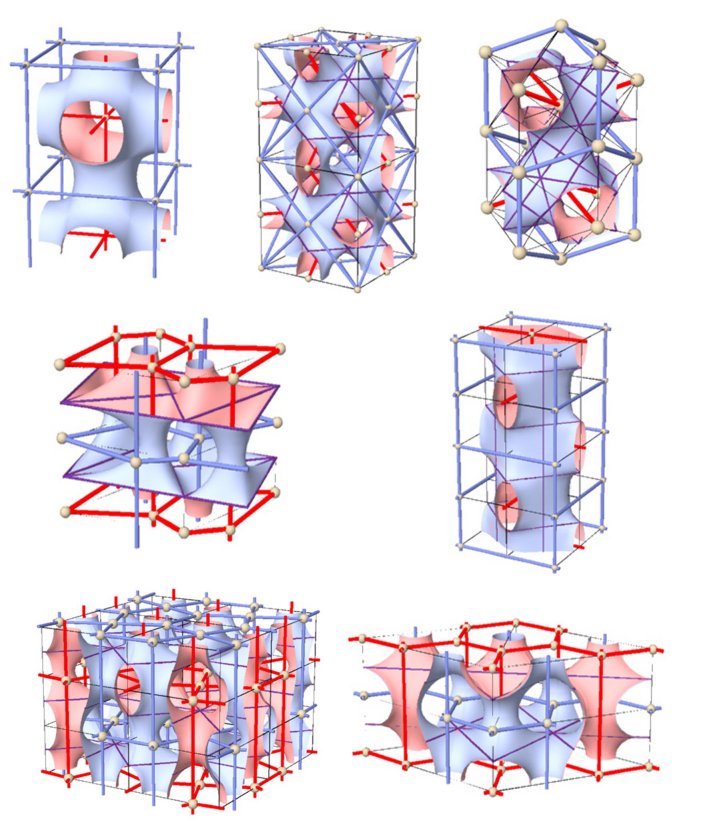
A new search method Finding the new self-dual network opened the door to revealing a completely new array of surface-partitions and the associated self-dual space networks, which characterize each of the two interwoven, complementary subspaces. In view of the last development, there is an advantage in shifting the gravity center of the research and pursuing the self-dual lattices pairs first. The new search method is built of several steps, and is partially based on the exhaustive method:
- 1:1 relation between edges of one
of the lattices to the faces of the dual.
- 1:1 relation between vertices of one
of the lattices to the packing cells of the dual, and vice versa.
Find the 2-manifold unit, which separates
the complementary dual lattices, in a manner that assures continuity and
smoothness over the boundaries of the E.P.R. and for the whole periodic
hyperbolic surface. It requires that its intersection with the bounding
reflection planes will be perpendicular to these planes all along the intersection
curve.
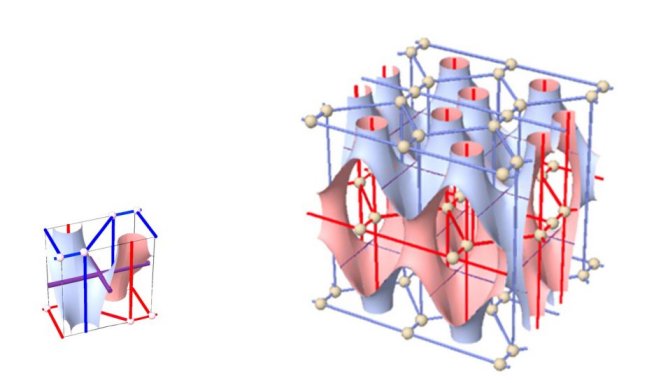
Figure 15 Self dual lattices
Figure 16 2-manifold
surface that subdivides
Conclusions Considering the apparent significance of the subject of dual lattice pairs and the subsequently related continuous Periodic Hyperbolic Surface-partitions, within the general theme of Space Subdivisions, it has never occupied a central position in a systematic and exhaustive research inquiry with these complementary spatial configurations. It should be noted that these configurations are the abstract idealized prototypes of all sponge structures, all labyrinth-like branching, infinite, enveloped, spaces and other natural phenomena, such as periodic filament configurations and continuous warped fields. They are an idealized geometric abstraction of continuous labyrinths, which shape the interiors of our modern mega-structures and our evolving urban down town environments. As of now it is clear that a general comprehensive notation system of space lattices is urgently required, as well as additional elaboration on the subject of "universal parameters" capable of capturing the nature and quality of the various ordered space lattices and related hyperbolic surfaces. It is clear also that any conspicuous
advance in this research direction is conditioned by a development of a
powerful and effective computerized generation and graphical representation
of these lattice-surface complexes, which will be based on an input of
a limited number of parameters on the simplex-E.P.R. level.
References: D.R.J. Chillingworth, Differential topology with a view to applications, Pitman Publishing London, San Francisco, Melbourne, 1976. Hilbert & Cohn-Vossen, Geometry and the imagination, Chelsea Publishing Company, New York, 1952. H.S.M. Coxeter, Regular Polytopes, Dover Publication Inc., New York, 1973 M. Burt, Spatial Arrangement and Polyhedra with Curved Surfaces and their Architectural Applications, Technion, Haifa, 1966. Peter Gay, The Crystalline State, Oliver & Boyd Edinburgh, 1972 M. Burt and A. Korren, Periodic hyperbolic surfaces and subdivision of 3-space, Hyperspace, vol. 3, #3, Japan, Dec. 1994. A. Korren, Periodic
2-manifolds surfaces, which divide the space into two identical subspaces,
Master thesis, Technion, Israel, 1993.
|