|
Symmetry transformations of polyphonic melody structure
From the aspect of symmetry analysis, the same symmetry transformations occur in the symmetry of counterpoint as in the symmetries of melodic structure, the only difference being that the former have an impact on the elements of one voice, transforming them into elements of a second voice. Without straying from the general subject we will examine only the symmetry analysis of two-part compositions. In this case the following analogous counterpoint isometric transformations occur:
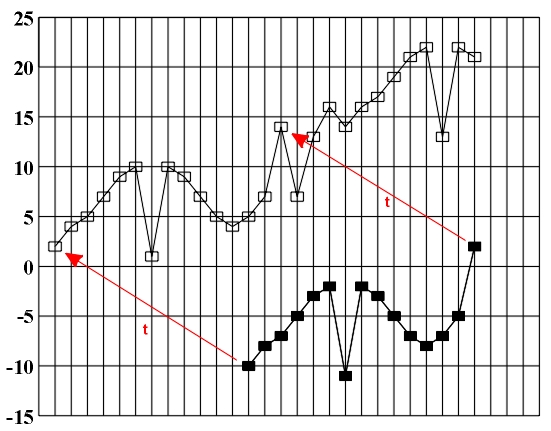
Figure 4.7 J. S. Bach, Two-part invention no. 4, an example of absolute imitation (translation tx,y).
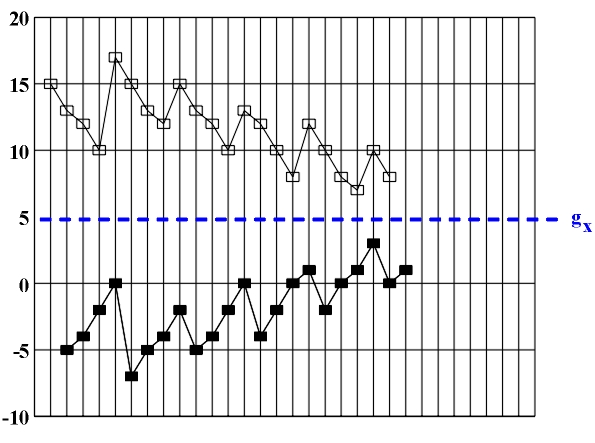
Figure 4.8 An example of inversion (glide reflection gx) (M. Tajcevic: Kontrapunkt, example 450, p. 152).
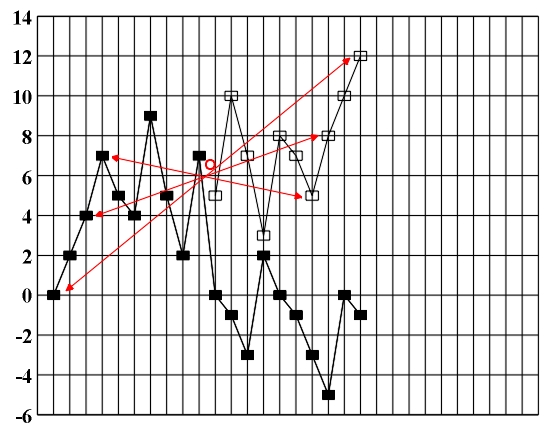
Figure 4.9 Crab imitation (central reflection O).
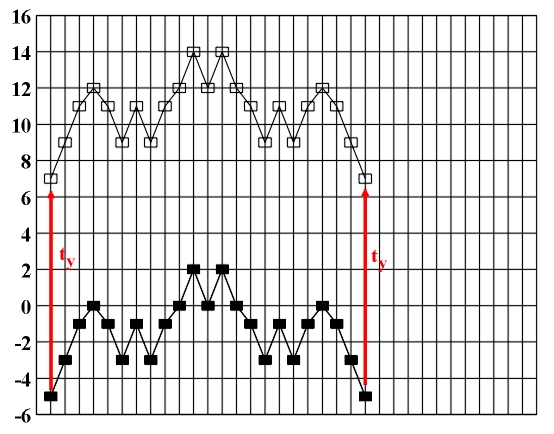
Figure 4.10 Retrograde shift (glide reflection gy) (Musical Encyclopedia 2, 1963, p.481). With this two specific new cases of the above mentioned transformations occur:
The first transformation (1a) brings about the appearance of a parallel melody, and the second (2a), the appearance of an anti-parallel melody in different voices.
Figure 4.11 B. Bartok, Microcosmos, Vol. 1, Six Unison Melodies, part VI - an example of unison imitation (translation ty) (Apagyi, 1989).
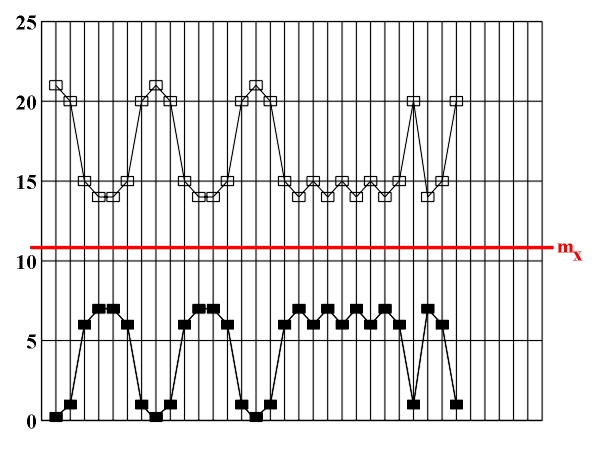
Figure 4.12 L. Papp, Leggiero. An example of anti-parallel imitation (mirror reflection mx) (Apagyi, 1989).
The symmetry analysis of polyphonic pieces may be further expanded
by a study of the counterpoint transformations of similarity,
counterpoint permutation equivalence, etc. Different aspects
of combining symmetry transformations of the rhythmic and melodic
structures within counterpoint (e.g., counterpoint augmentation or
diminishing) also occur.
|