Definitions of symmetry

In modern science, symmetry is defined in two ways:

1. as a regular arrangement of equivalent elements (Senechal, 1989);

2. as the invariance of one object under the action of transformations (Rosen, 1989).

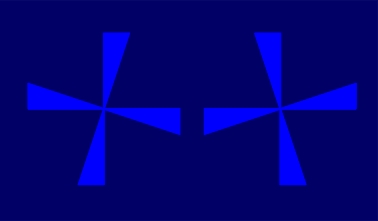
Based on the first definition, symmetry is the result of the fact that each of the equivalent elements is surrounded by other equivalent elements in the same manner. This definition could be treated as inductive or generic, as it points to the genesis of symmetric objects. We begin with one element and surround it with other equivalent elements. Then, in keeping with the same principle, we repeat the procedure by applying it to each subsequent element.

By performing this procedure in the opposite direction, we can divide any symmetric figure into two or more equivalent parts arranged in the same manner. Any figure not permitting such a division is asymmetric. The genesis of a symmetric figure is illustrated in the following example. By rotating an asymmetric figure by 90^° a symmetric figure (rosette with the symmetry group C₄) is obtained (Fig. 1.1).

Figure 1.1. The derivation of rosette C₄.

The second definition which can be classified as deductive, describes the symmetry of an object as its resistance (invariance) with regard to a certain change t (non-identical transformation t, i.e., a transformation that does not copy each point in itself). This means that the complete figure f, after transforming it by transformation t, remains unchanged: t(f)=f. In this case, transformation t is the symmetry of figure f. If there is no such non-identical transformation t, the figure f is asymmetric. For example, each rotation of rosette (Fig. 1.1) around its center for 90^° , 180^° and 270^° transforms it into itself (leaves it unchanged), so that these rotations are the symmetries of this rosette.

Because of the significance of all the principles of conservation (of matter, energy, etc.) in the sciences, this definition is very frequently used. In the natural sciences, especially physics, the concept of symmetry is in many cases reduced to the motion of a solid without deformation.

We can draw several questions from the above-mentioned definitions. What means the term "regular arrangement of equivalent elements", i.e., what are the transformations whose action does not change a symmetric figure? The term ëquivalent elements" is sufficiently general, as it can relate to congruent (equal) elements, similar (proportional, i.e., proportionally augmented or diminished) elements, or elements which are related based on any other previously given criterion (a relation of equivalence). The expression "regular arrangement" means that the same rule is applied to each equivalent element. From this we can deduce the existence of non-identical transformation, symmetry of the figure considered, which preserves the figure unchanged and transforms each element of the figure which is surrounded by other elements according to the same rule. From this follows the mutual equivalence of the two definitions cited.

Depending on the type of equivalence of the elements, the corresponding transformation can be congruency (isometry), similarity (proportion), or some other type of transformation. This permits the possibility of classifying symmetries based on the type of transformation. Thus we can differentiate between isometric symmetry, symmetry of similarity, etc.