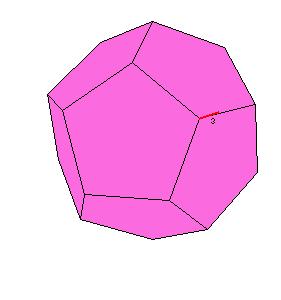
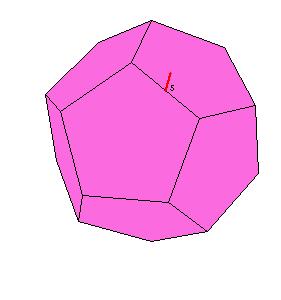
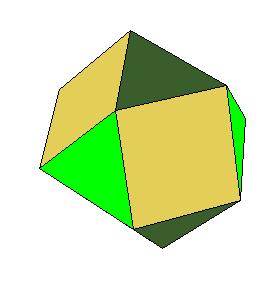
3D illustrations to Chapter 8 of Cromwell's Polyhedra
Izidor Hafner
Tomislav Zitko
Faculty of Electrical Engineering, University of Ljubljana
Trzaska 25
, 1000 Ljubljana
, Slovenia
e-mail: izidor.hafner@fe.uni-lj.si
One way to improve teaching of stereometry is to give 3D illustrations to well known textbooks.
As an example let us take Chapter 8 of Cromwell's Polyhedra. Chapter 8 is dealing with symmetry.
Systems of rotational symmetry
Cyclic symmetry
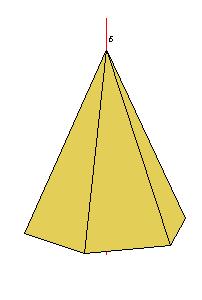
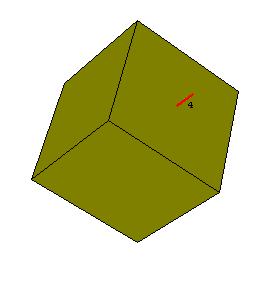
|
| Figure 8.1. A rotation axis in a cyclic system
|
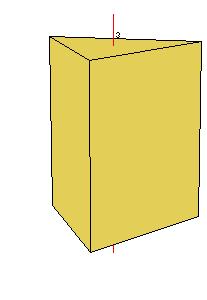
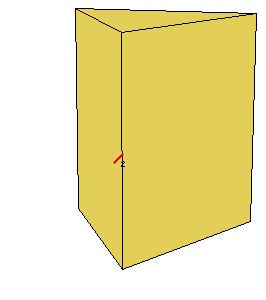
Dihedral symmetry
| 
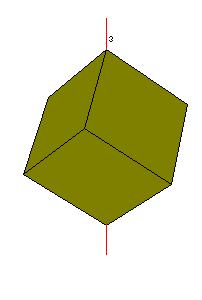
|
| Figure 8.2. Principal and secondary axes in a dihedral system
|
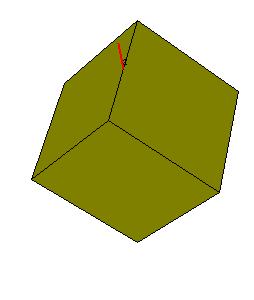
| 
|
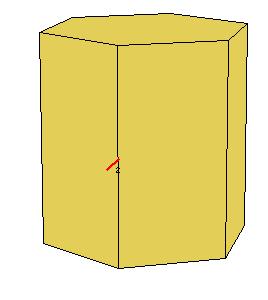
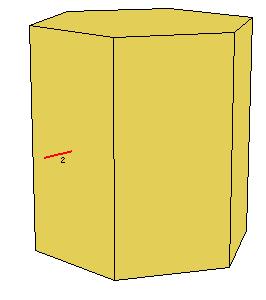
| Figure 8.3. When n is even, the secondary axes in Dn can be separated into two kinds
|
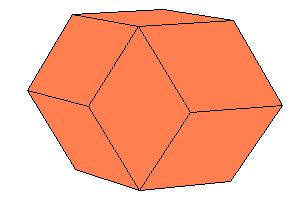
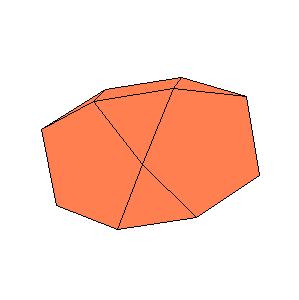
| 
|
| Figure 8.4. Polyhedra with D2 symmetry.
|
Tetrahedral symmetry
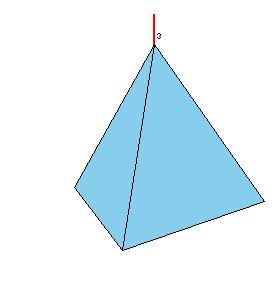
| 
|
| Figure 8.5. Rotation axes in the tetrahedral system.
|
Octahedral symmetry
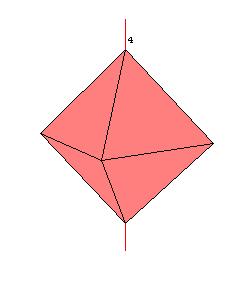
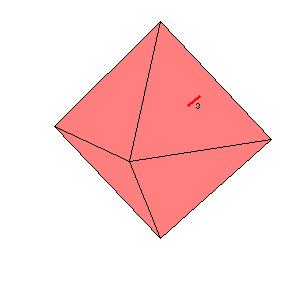
| 
| 
|
| Figure 8.6. Rotation axes in the octahedral system.
|
Icosahedral Symmetry
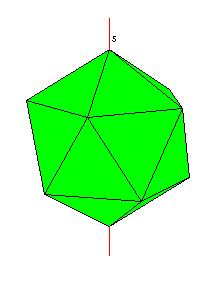
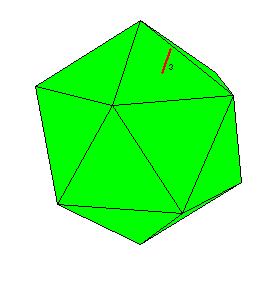
| 
| 
|
| Figure 8.7. Rotation axes in the icosahedral system.
|
Reflection symmetry
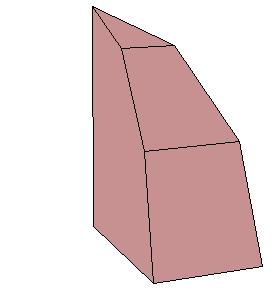
|
| Figure 8.9. A polyhedron with bilateral symmetry.
|
Prismatic symmetry types

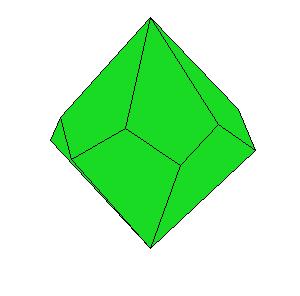
| 
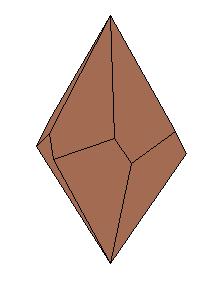
| 
|
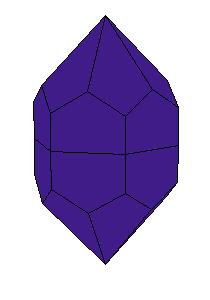
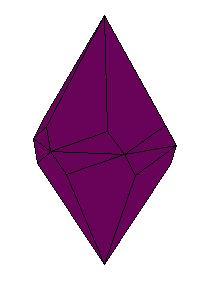
| 
|
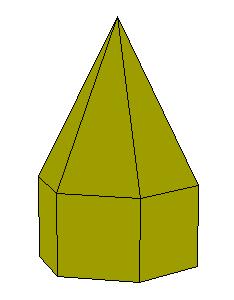
| 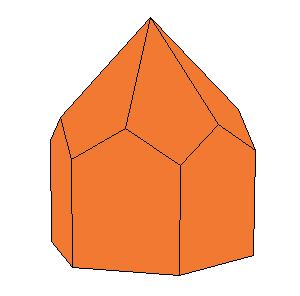
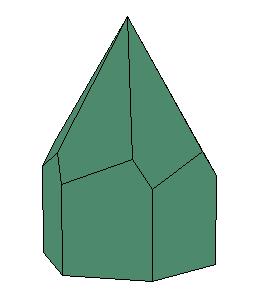
| 
|
| Figure 8.10. Polyhedra with prismatic symmetry.
|
Symmetry type Dnh.
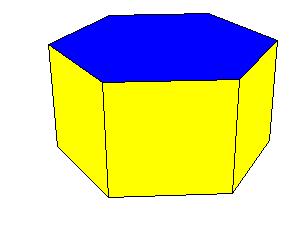
|
| Figure 8.11.
|
Symmetry type Dnv.
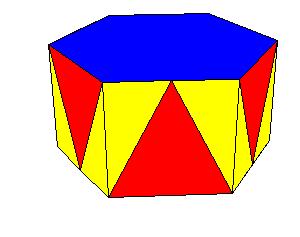
|
| Figure 8.12.
|
Symmetry type Dn.
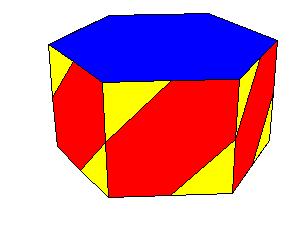
|
| Figure 8.13.
|
Symmetry type Cnv
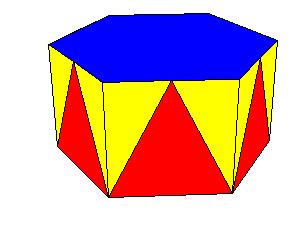
|
| Figure 8.14.
|
Symmetry type Cnh
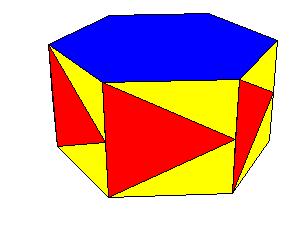
|
| Figure 8.15.
|
Symmetry type Cn.
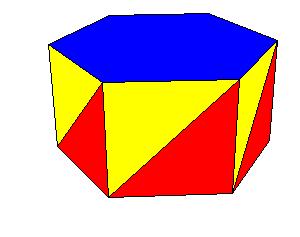
|
| Figure 8.16.
|
Compound symmetry and the S2n symmetry type
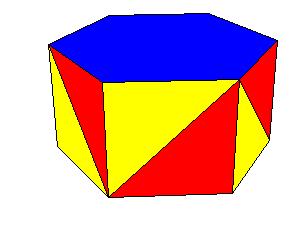
|
| Figure 8.17.
|
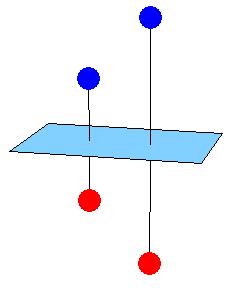
| 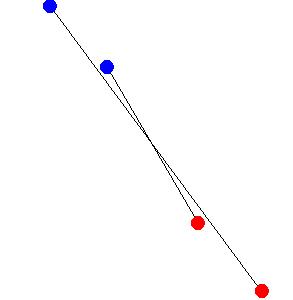
|
| reflection in a plane
| reflection in a point
|
| Figure 8.20.
|
Cubic symmetry types
Symmetry type Oh.
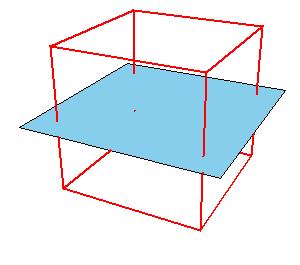
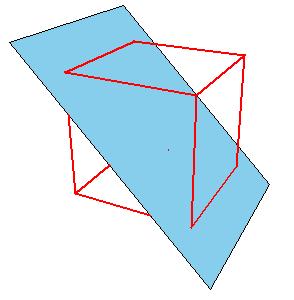
| 
|
| Figure 8.21. The reflection planes of a cube.
|
Symmetry type O
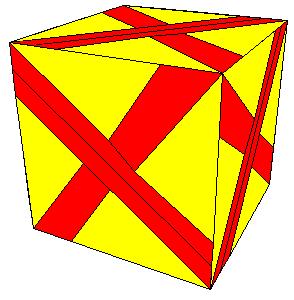
|
| Figure 8.22.
|
Symmetry type Th.

|
| Figure 8.23.
|
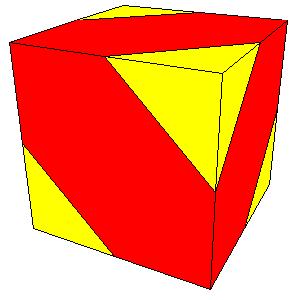
|
| Figure 8.25.
|
Symmetry type T
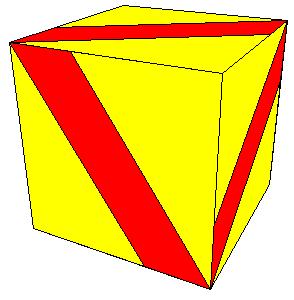
|
| Figure 8.26.
|
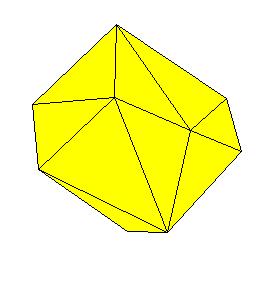
|
| Figure 2.27.
|
Some examples
The cube has octahedral rotational symmetry.
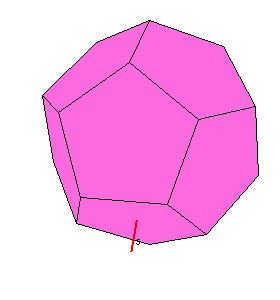
The dodecahedron has icosahedral rotational symmetry.
We get examples of tetrahedral symmetry by colouring polyhedra with octahedral and icosahedral symmetry.
References
[1] P. R. Cromwell, Polyhedra, Cambridge University Press 1997.
[2] Martin Kraus' Live3D applet