|
Paulus Gerdes Mozambican Ethnomathematics
Research Centre, C.P. 915,
Abstract The paper presents an update on strip patterns found on twill-plaited handbags and baskets made by Tonga artisans, mostly women. It includes a catalogue of 58 new strip patterns that appear on sipatsi collected between August and December 31, 2002. All seven symmetry classes are represented. Attention is drawn to two particular types of strip patterns characterised by special plaiting structures, that we call single and double. Introduction Gitonga is one of the languages spoken in the Inhambane province in Southeast Mozambique. It is the main language of the population living in the coastal districts of Inhambane, Jangamo, Maxixe and Morrumbene. Gitonga is the mother tongue of about 170.000 people. Because of their beauty and utility, baskets made by Tonga weavers, who are mostly women, are among the most appreciated products of Mozambican craftwork. The baskets of the highest quality and with most variation in their design are produced on the peninsula of Linga-Linga, almost only reachable by boat. The peninsula lies in the district of Morrumbene, about 500 km to the Northeast of the country’s capital Maputo. Some baskets are commercialised at nearby markets but others find their way directly to the markets of the capital and of the neighbouring countries Swaziland and South Africa. The books (Gerdes & Bulafo 1994) and (Gerdes 2003) analyse Tonga handbags called gipatsi (Plural: sipatsi). To produce these handbags the artisans use mostly as basic weave the ‘over two, under two’ twill (notation: 2/2). By introducing some systematic changes in the weave along bands of the texture, they create attractive strip patterns (for an introduction see Gerdes 1998, chapter 1 or Gerdes 1999, pp. 87, 140-143). Photographs 1 and 2 present two examples of sipatsi. In one weaving direction all the strands are coloured, while in the opposite direction all strands maintain their natural colour (white-yellow). In the last years some strip patterns found their way to other plaited objects like hats and larger bags (see the examples in Photographs 3 and 4). Among other new phenomena we may note the colour transformation of strip patterns (see Gerdes 2002) and the invention of plane patterns on sipatsi (see Gerdes 2003a). 
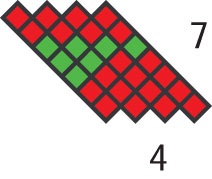
Photograph 1 Photograph 2 Photograph 3 Photograph 4 The Tonga weavers are also very creative in inventing new strip patterns. Whereas the catalogue in (Gerdes & Bulafo 1994) included 96 different strip patterns (collected in the period 1977-1993), the catalogue in (Gerdes 2003) includes 362 different strip patterns collected until July 2002. This paper presents 58 new strip patterns that appear on sipatsi collected between August and December 31, 2002. Representation of strip patterns Figure 1 illustrates part of a gipatsi. In the illustration, the darker strands, in fact, are purple, dark green, or, dark blue (see the photographs). The use of different colours makes sipatsi more attractive. For the weavers of sipatsi, the beauty of the ornaments resides principally in the quality of the contrast generated by the plaiting of sets of strands of natural colour ("white-yellow" strands) with coloured strands. For this reason, we may represent all coloured strands with the same colour. 
Figure 2 
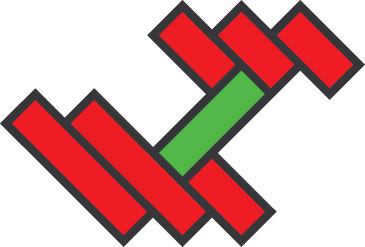
Figure 3 As the direction of individual strands is (almost) not visible, we may remove them from the illustration. In this way, the decorated strip, of which Figure 2 shows only a portion, is transformed into the strip of Figure 3. For any gipatsi, on the left and right of the motif of an ornamental strip, there is always another exemplar of the same motif. In this sense, one may consider an ornamental strip on a gipatsi as infinite. Consequently, one may consider the part of the strip illustrated on a piece of (planar) paper as representing the whole strip that extends infinitely to the left and to the right, always repeating at fixed distance the same decorative motif. A strip of this nature is called a one-dimensional strip pattern. Strip patterns that derive from ornamental strips on sipatsi we will call gipatsi-patterns. We may say that the toothed parallelogram in Figure 4 generates the gipatsi-pattern in Figure 3. In our example, the toothed parallelogram has the dimensions 4 by 7, that is, 4 contiguous oblique strands, each composed of 7 unit squares (see Figure 5). The sides of the unit square are equal to the width of the strand. 
Figure 4 Figure 5 We will call the first dimension the period of the decorative motif and the second dimension its diagonal height. In the given example, we have 4 as the period. In other words, the period indicates how many coloured strands are necessary to generate the decorative motif. For each pattern in the complementary catalogue of gipatsi-patterns we will indicate a generating toothed parallelogram. The gipatsi-patterns will appear in increasing order of their period. Patterns of the same period come in increasing order of their diagonal heights. A gipatsi-pattern will be represented by p×h [n], being p its period, h its diagonal height and n its order among the gipatsi-patterns with dimensions p×h. For instance, the pattern with the code 2×9 [4] refers to the fourth pattern with dimensions 2 by 9. Figure 6 presents the 58 new gipatsi-patterns.
Symmetries Among the 58 gipatsi-patterns, all seven (one-colour) one-dimensional symmetry classes are represented. For an introduction to these symmetry classes and explanation of the international notation, see e.g. Washburn & Crowe. Table 1 gives the distribution of the additional gipatsi-patterns by symmetry classes.
Table 1 Subjacent symmetries In several cases, the symmetries become stronger if one considers only the natural coloured parts of the gipatsi-patterns. Figures 7 and 8 illustrate two examples of gipatsi-patterns and their subjacent strip patterns. Table 2 presents the distribution of the subjacent patterns by symmetry class. 
Figure 7a
Figure 8a
Figure 8b
Table 2
Gipatsi-patterns with a single plaiting structure Tonga basket weavers often prefer a type of strip pattern characterised by a particular plaiting structure. Figure 9 presents a small part of a decorated band. The coloured strand passes exactly once under a set of contiguous natural strands (in this example, three contiguous natural strands). Similarly, Figure 10 presents another part of a decorated strip. The natural, uncoloured strand goes over exactly one set of contiguous coloured strands, whereas in the example presented in Figure 11 the natural strand passes over two sets of contiguous coloured strands. 
Figure 9 Figure 10 Figure 11 If along a decorated band each of the coloured strands passes exactly once under a set of natural strands, the decorated strip may be called C-single. Figure 12 presents an example of a C-single strip pattern. 
Figure 12 If along a decorated band each of the natural strands passes exactly once over a set of coloured strands, the decorated strip may be called N-single. Figure 13 presents an example of a N-single strip pattern. 
Figure 13 A plaited strip that is both C-single and N-single may be called single. Thirty of the 58 gipatsi-patterns in Figure 6 are single (# 365, 366, 369-373, 377-379, 384, 385, 387, 394-398, 403-405, 407-410, 412, 414, 416, 418, 419). Three are only C-single (# 368, 386, 399) and none is only N-single. All eleven gipatsi-patterns that belong to the pmm2 symmetry class are single. Gipatsi-patterns with a double plaiting structure Eighteen of the 58 gipatsi-patterns in Figure 6 are double. This means that along the plaited band each coloured strand passes exactly twice under sets of contiguous natural strands and each natural strand passes exactly twice over sets of contiguous coloured strands (# 363, 364, 374-376, 380-383, 388, 390-393, 400, 402, 406, 415). Most of them have pm11 symmetry, two display a pma2 symmetry (# 383, 406), one has a p1a1 symmetry (# 393), while three are only invariant under translation (p111) (# 363, 364, 415). References ___ (1999) Geometry from Africa: Mathematical and Educational Explorations, Washington DC: The Mathematical Association of America ___ (2002) Colour transformation of strip patterns in Tonga basketry (Mozambique), Maputo: Ethnomathematics Research Centre, 20 pp. ___ (2003) Sipatsi: Cestaria e Geometria na Cultura Tonga de Inhambane, Maputo: Moçambique Editora, 178 pp. (in press) ___ (2003a) Exploring plaited plane patterns among the Tonga in Inhambane (Mozambique), Symmetry: Culture and Science, Budapest (in press) ___Gerdes, Paulus and Bulafo, Gildo (1994) Sipatsi: Technology, Art and Geometry in Inhambane, Maputo: Universidade Pedagógica ___Washburn,
Dorothy K. and Crowe, Donald W. (1988) Symmetries of Culture. Theory
and Practice of Plane Pattern Analysis, Seattle: University of Washington
Press
|