|
Mwani colour inversion, symmetry and cycle matrices
Paulus Gerdes Research Centre for Mathematics, Culture and Education, C.P. 915,
Kimwani is a language spoken in the coastal areas of the Cabo Delgado province
in the extreme Northeast of Mozambique.
It is closely related to or may be considered one of the southern
dialects of the Swahili language (Firmino, 1989, p. 11; Bento, 1993). The so-called ‘beach language’ is also
related to the Makhuwa language (Medeiros, 1997, p. 59). The Mwani result from the secular
bio-cultural miscegenation of already ‘Swahilised’ Africans coming from
coastal zones and islands further north and local African populations
(Makhuwa, Makonde,
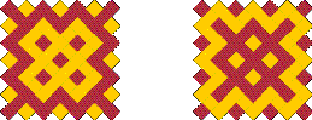
Detail of a luanvi Figure 1
Rectangular mats, called luanvi (Bento, 1993, footnote 297),
are made from brightly dyed palm fibre by sewing long plaited bands together
(see the example in Figure 1). The
mats are laid down on the floor to eat, talk, sleep or rest upon, or hung on
the walls as decoration. One-colour
plain strips separate the decorated bands from each other. Women weave the two-colour ornamental
bands, whereas men make the one-colour bands and sew the plaited bands
together (Dias & Dias, 1965, p. 152; Dias & Dias, 1970, p. 112). Mwani
colour inversion The strands make angles of
45o with the border of the band; when the end of a plaited line is
reached, the strands are bent back to be worked in the opposite direction
(see Figure 2).
Figure 2 As the strands are very thin
with a width of only slightly more than 1 mm, the women plait their decorated
bands with a double thickness of strands.
Most two-colour ornamental bands are woven in such a way that
dark-coloured dyed strands and natural strands alternate in both weaving
directions. As a consequence of this
plaiting process the visual image of a design motif on the front side is
normally different from the visual image on the backside of the mat: (1) Where a dark strand crosses with a dark
strand, we have a dark spot (unit square) on both sides; (2) Where a natural strand crosses with a
natural strand, we have a naturally coloured spot on both sides; (3) Where a dark strand crosses with a natural
strand, we have on one side a dark unit square but on the other side a
natural unit square; the colours have been reversed.
Figure 3 Let us call this particular,
partial colour inversion the Mwani
colour inversion: one half of the
unit squares has the same colour on both sides of the mat (see the coloured
unit squares in Figure 3), whereas the other half of the unit squares (white
in Figure 3) have opposite colours on both sides of the mat. Figure 4 presents examples.
Front side
Backside a
Backside b Figure 4 The Mwani colour inversion
is independent from the particular arrangement (or twill) of the strands used
to produce the design element on the front side. Figure 5 presents the twill of a Mwani
motif; and Figure 6 its visual image.
Any other twill that produces the same visual image, generates also
the same reverse image on the backside of the mat (Figure 7).
Weaving texture / twill Figure 5
Visual image of the strip pattern (front side) Figure 6
Visual image of the strip
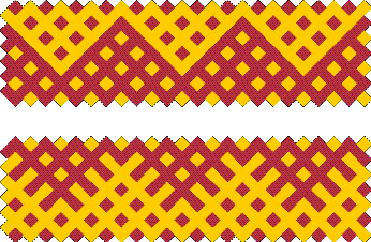
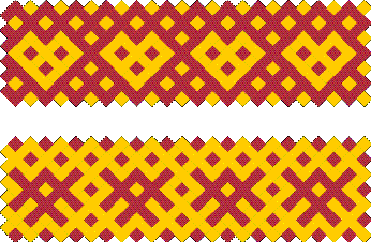
pattern (backside) Figure 7 Symmetries If we do not take into account the border area where the strands are reversed, the front and back image of any decorated strip display the same symmetries. Not considering the trivial symmetry class p111, whereby a strip is only invariant under a translation, the Mwani women use all symmetry classes of strip patterns. Figure 8 presents examples (front and back sides).
1) Class pmm2
2) Class pma2
3) Class pm11
4) Class p1m1
5) Class p112
6) Class p1a1 (‘footstep’ symmetry)
Figure 8
In each case, the backside is shown as ‘looking through the strip;’ in reality, the human eye sees the strip at the backside up side down, or interchanging left and right.
Moreover, some decorated strips display two-colour symmetries. In other words, there exists a rotation, reflection or glide-reflection that interchanges the colours of the strip. Figure 9 presents examples of class pma’2’.
a
b Figure 9
Mwani women found exceptional cases whereby the backside image is not only the Mwani-colour-inverse, but also the ‘real’ colour inverse of the front side. Figure 10 presents examples of class pmm2.
a
b Figure 10
On other Mwani mats, their colour inversion reverses the direction, when one ‘looks through.’ Looking at the backside, the direction, however, is the same; only the colours are reversed. Figure 11 presents an example of symmetry class p1m1.
Figure 11
Mwani women succeeded in constructing strip patterns that look the same on both sides of the mat. Figure 12 presents an example. ‘Looking through the mat’ the direction of the ‘arrows’ is reversed; to the human eye the strip looks the same on both sides of the mat.
Figure 12
Cycle matrix structures
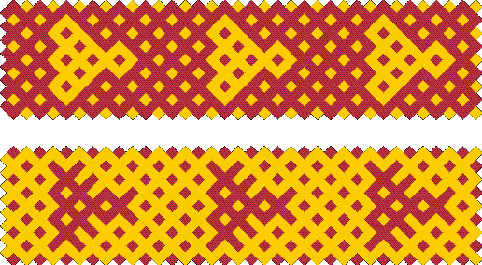
Figure 13a displays part of the front and backside of a Mwani mat. Figure 13b presents the same design motifs on another mat.
a
b Figure 13
Surprisingly, where the first design element corresponds to the structure of a negative cycle matrix of dimensions 4 by 4, its Mwani colour inversion corresponds to the structure of a positive cycle matrix of the same dimensions (cf. Gerdes, 2002, 2007). Figure 14 presents the general structure of positive and negative cycle matrices of dimensions 4x4 and period 2. In the case of the negative cycle matrix, it is composed of two cycles of alternating numbers. The multiplication of cycle matrices follows the ‘rule of signs’: negative times negative is positive, etc.
Positive cyle matrix a
Negative cycle matrix b Figure 14
Figure 15 shows how these cycle matrix structures appear on the Mwani strips in Figure 13.
Figure 15
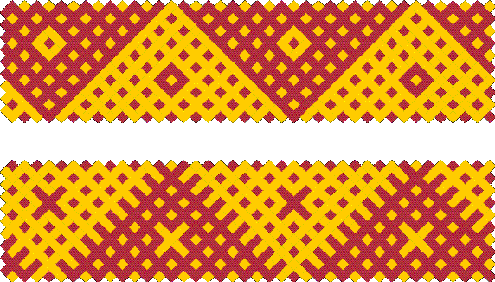
The image on the front and backside corresponds to the negative and the positive cycle matrix structure of dimensions 4x4. Similarly, the structures of negative and positive cycle matrices of other even dimensions can be plaited in the Mwani way, and each time the negative structure on the front side of a mat produces through the Mwani colour inversion the corresponding positive structure on the backside. Moreover, the same is possible for the structures of cycle matrices of odd dimensions. Figure 16 displays the case of cycle matrices of dimensions 3x3 and 5x5.
Plaited designs corresponding to the positive and negative cycle structures of dimensions 3x3 a
Plaited designs corresponding to the positive and negative cycle structures of dimensions 5x5 b Figure 16
In the case of the positive cycle structure, all unit squares that change colour under the Mwani colour inversion (see the ‘sand’ coloured unit squares in Figure 17) have the same light colour on the front side; on the backside they are all dark coloured.
Figure 17
Surprising relationships
In earlier studies I formulated the hypothesis of how the basic ideas for starting the sona drawing tradition from East Angola and neighbouring areas of Congo and Zambia, could have been derived from mat weaving (cf. Gerdes, 2006). The mathematical study of sona led me to the discovery of Lunda-designs (see Gerdes, 1999) and Liki-designs. On their turn, the study of Liki-designs led to the discovery of cycle matrices and their beautiful visual-geometric properties (cf. Gerdes, 2002, 2007). Now, in this paper, we see how the Mwani women’s way of producing decorated bands for their mats implies a special, partial colour inversion that we call the Mwani colour inversion. Surprisingly, this Mwani colour inversion is intrinsically related to the inversion of positive into negative cycle matrix structures of any dimensions.
The Mwani colour inversion in a wider cultural context
Mats similar to the ones produced by the Mwani, are produced in other Swahili(sed) cultures. Trowell (1960, Plate XIII) presents 12 examples of plaited decorated bands on mats from Zanzibar, where dark dyed and natural strips are alternating (cf. Gerdes, 1999, 142-146). On one band the cycle matrix structure of dimensions 2×2 appears. Figure 18 shows the visual images of the front and backside of two bands in the Trowell collection.
a
b Figure 18
The Mwani decorated band in Figure 9a is also in the Trowel’s Zanzibar collection. The Mwani band displayed in Figure 19a has the same design element as a band in Trowell’s collection (see the front and backside display in Figure 19b).
Detail of a Mwani mat a
Visual image of both sides of a mat from Zanzibar b Figure 19
A decorated band reported from Mafia Island displays also the same design element (see Figure 20). Freyvogel (1961) presents a collection of plaited mats from the Ulanga District, also in Tanzania.
A decorated band from Mafia Island Figure 20
The postage stamp reproduced in Figure 21 shows that mats similar to the luanvi are used at the Comoro Islands.
Postage stamp from the Comoro Islands Figure 21
The Mwani and Swahili tradition of plaiting decorated bands with a rich repertoire of design elements and design combination deserves further study. For instance, Figure 22 presents an example of a Mwani decorated band with a combination of two design elements. Figure 23 displays both sides of the design combination.
Figure 22
Figure 23
Also the use of the Mwani colour inversion in other cultural contexts, including the transformation of plane patterns, deserves further study. In (Gerdes, 2003) some examples from Tonga women in Inhambane Province in Southeast Mozambique are presented.
In Gerdes (1998, 2003) other examples are presented of female cultural activities in Southern Africa, wherein artistic-geometric considerations play a role (see also the annotated bibliography Gerdes & Djebbar, 2007).
References:
Bento, Carlos Lopes (1993), As Ilhas de Querimba ou de Cabo Delgado. Situação colonial, resistências e mudança, Universidade Técnica de Lisboa (Ph.D. thesis) (http://br.geocities.com/quirimbaspemba).
Dias, Jorge & Dias, Margot (1964), Esteiras, in: Dias, Jorge & Dias, Margot, Os Macondes de Moçambique, Junta de Investigações do Ultramar, Lisboa, Vol. 2, 150 – 159.
Dias, Jorge & Dias, Margot (c. 1970), Moçambique – Cestaria e esteiraria, A arte popular em Portugal, ilhas adjacentes e ultramar, Lisboa, No. 30, 97 – 122.
Firmino, Gregório (Ed.) (1989), I Seminário sobre a Padronização da Ortografia de Línguas Moçambicanas, INDE-UEM, Maputo.
Freyvogel, Thierry A. (1961), A collection of plaited mats from the Ulanga District of Tanganyika, Tanganyika Notes and Records, Dar-es-Salaam, No. 57, 139-148.
Gerdes, Paulus (1998), Women, Art and Geometry in Southern Africa, Africa World Press, Lawrenceville NJ. ___ (1999), Geometry from Africa: Mathematical and Educational Explorations, The Mathematical Association of America, Washington DC. ___ (2002), From Liki-designs to cycle matrices: The discovery of attractive new symmetries, Visual Mathematics, 4(1) (http://members.tripod.com/vismath7/gerd/) ___ (2003), Sipatsi: Cestaria e Geometria na Cultura Tonga de Inhambane, Moçambique Editora, Maputo. ___ (2006), Sona Geometry from Angola. Mathematics of an African Tradition, Polimetrica International Science Publishers, Monza ___ (2007), Adventures in the World of Matrices, Nova Science Publishers, New York.
Gerdes, Paulus & Djebbar, Ahmed (2007), Mathematics in African History and Cultures: An annotated Bibliography, LULU.com, New York.
Medeiros, Eduardo da Conceição (1997), História de Cabo Delgado e do Niassa (c. 1836 – 1929), Cooperação Suiça, Maputo.
Trowell, Margaret (1960), African Design, Praeger, New York.
Sources of illustrations:
All drawings were made and all photographs were taken by the author, with the exception of the postage stamp from the Comoro Islands.
The mats were acquired or photographed in 1998 Figures 5, 6, 7: Drawn after a design on a mat acquired by the author in 1998. Figure 8: Drawn after photographs taken in 2003 (pm11, p112), 2004 (p1m1), and 2007 (pmm2, pma2, p1a1). Figure 9: Drawn after photographs in Dias & Dias (1970, p. 118) and Bento (1971[available on the internet]). Figure 10: Drawn after photographs taken in 2007 (a) and 1998 (b) [the last one appears also in Dias & Dias, 1970, p. 119]. Figures 11 and 12: Drawn after photographs taken in 2007. Figure 13: Photographs taken in 2005 and 2007. Figure 14: Reproduced from Gerdes (2007). Figure 15: Drawn after photographs taken in 2005 and 2007. Figure 18: Drawn after photographs taken in Trowell (1960, Plate XIII). Figure 19a: Photograph taken in 2007. Figure 19b: Drawn after photographs taken in Trowell (1960, Plate XIII). Figure 20: Drawn after a photograph on the web. Figure 21: Photograph on the web. Figure 22: Photograph taken in 1998. Figure 23: Drawn after a photograph taken in 1998.
|