Geometrical, Perceptual, and Cultural Perspectives on Figure/Ground Differences in Bakuba Pattern
Donald W. Crowe,
Department of Mathematics
University of Wisconsin-Madison
Madison, WI 53706
E-mail: crowe@math.wisc.edu
Dorothy K. Washburn
Laboratory of Anthropology
Museum of New Mexico
Santa Fe, NM 87504
E-mail: dkwashburn@worldnet.att.net
Abstract
Two tabletops carved by a Bakuba wood-carver reveal a surprising duality. Although the carvings at first glance appear completely different, closer attention shows that the carved portion of each is exactly the uncarved portion of the other. Hence, in a certain sense, they have exactly the same symmetries. We discuss the cultural insights suggested and supported by this observation.
1. Introduction
In 1985 Dorothy Washburn visited a number of villages throughout south central Zaire (now the Democratic Republic of Congo) and studied the pattern symmetries on raffia cloths made by tribes in the Bakuba Kingdom [14]. As a memento of that trip she commissioned the carving of two small tabletops (Figures 1, 2). The carver, Art Pembe of Kananga, chose the designs. Washburn’s only stipulation was that they should be from the Bakuba tradition. It was thus very interesting that, although he carved two designs the Bakuba consider to be distinctly different named entities―Mamanye and Mikobi Ngoma ―they are, from the perspectives of geometry and perceptual psychology, figure/ground reversals of the same symmetrical pattern. This identity in symmetry is obscured by the cultural overlay of the Bakuba peoples’ aesthetic style whereby contrasting colors, lines and textures in the pattern are manipulated causing the Mamanye pattern to be of symmetry class p4g while Mikobi Ngoma is of class pgg. In fact, as presented, the patterns on these tables have symmetry types cmm and p2 respectively. This discrepancy in classification will be discussed along with Figures 1a and 2a in Section 2.

Figure 1: Mamanye pattern on tabletop carved
by Bakuba woodcarver Art Pembe.

Figure 2: Mikobi Ngoma pattern on tabletop
carved by Bakuba woodcarver Art Pembe.
Note that the textured portion of each is the blank portion of the other.
The early research team of Emil Torday and T.A. Joyce from the British Museum wrote extensively on the patterns used by the Bushong, the ruling class among the Bakuba [12]. They recognized that the same name was often given to two designs that appeared quite different, and based their assessment of the patterns on the motifs, rather than on the layout of the design, showing how a repertoire of 17 motifs was used to generate a number of different named designs. In another book [11: 164-165] Torday relates a story from central Africa that illustrates this emphasis on motifs: "Sitting down I took out an old number of the Graphic and began to inspect the pictures with much deliberation. Holding it so that they were visible to others too, I noticed that the crowd gradually crept nearer and nearer, till at last they began to make audible comments upon what
they saw.― Singularly enough, they did not seem to take in the picture as a whole, but recognized first a face, then a hat, then a stick, till at last they exclaimed, ‘Why, it’s a man.’”In contrast, in this paper we explore how more global mathematical and perceptual perspectives can focus our attention on features of the patterns that appear fundamental to the Bakuba aesthetic system. A geometrical classification [16] highlights the cultural preferences for certain symmetries within the range of possibilities open to patternmakers from the several tribes in the Bakuba Kingdom. A perceptual examination clarifies the subtle but deliberate ways that manipulation of the basic pattern geometry by contrasting colors, lines, and textures creates figure/ground reversals that enable the production of variations on the basic geometric structure, several of which are perceived as different, named patterns.
2. Geometrical Perspectives
At the outset, it must be recognized that culturally-derived one- and two-dimensional patterns (as opposed to those generated by a computer program or some other random pattern generator untainted by cultural input) are, by the nature of the objects on which they appear, truncated versions of infinite patterns that exist theoretically on a planar surface. In the case of the carved tabletops that are the subject of this study, the two-dimensional patterns in Figures 1 and 2 are truncated by the edges of the small tables, so that only a few repeats are visible. In these and most other cases the viewer is able to successfully identify the symmetry of the pattern, although this truncation that limits the number of pattern repeats sometimes makes it more difficult to detect the presence of "mistakes" in the layout of the pattern.
For example, in Figure 1 careful inspection reveals "mistaken" placement of the two central chevrons along the bottom row of the pattern. There are also "mistakes" in the striations that indicate over-under relations in Figure 2. Typically found along the borders where the pattern maker begins to lay out the pattern, these irregularities are found on many Bakuba patterns. However, in other examples, such as the cloth shown in Figure 3, there are irregularities throughout. In that cloth there are twelve places (in 3 rows of 4 each) that at first appear to be local centers of fourfold rotation. But in fact only five of these are fourfold centers. Three others are centers of twofold rotation, and the remaining four are neither. Indeed, a field interview study investigating asymmetry in turn of the century raffia patterns [14:77ff.] revealed that a significant amount of variation in motif shape and pattern structure is quite acceptable within each named pattern category.

Figure 3: Raffia cloth Mikobi Ngoma pattern, with many "mistakes", especially in lower left.
(Cat. #AF 1469, University Museum,University of Pennsylvania. Obtained by Frobenius, 1906)
A related problem is that the layouts themselves are skewed in such a way that the rectangular grids that are the basis for the p4g and pgg patterns have been effectively "stretched" so that the right angles of the squares are not preserved although the sides of the squares remain parallel. Known mathematically as "affine transformations" (see [5] for a non-technical discussion), this kind of projection is highly characteristic of some art styles, such as Japanese representational painting prior to the 15th century where objects in scenes appear "tilted" up in the picture plane. Examination of Bakuba designs carved on cups and boxes as well as sewn on raffia cloth reveal that the affine transformations such as in the two patterns in Figures 1 and 2 do not appear consistently as they do in some art styles where they are one of the characterizing features. For example, Figures 4a and 4b illustrate two cups carved with identical designs. However, while the 90º angles are preserved in the design on the left cup, they are not preserved on the design in the right cup. Washburn [14] investigated the perceived difference between a diamond layout, cmm, and a square layout, p4m by showing patternmakers computer-generated patterns of these two symmetries, one essentially a skewed version of the other. While these patterns are distinguishable from one another by symmetry considerations, informants grouped them together, focusing instead on the similar way the shapes in both patterns are arranged along the linear "streets" (empty paths that separate the rows of squares and diamonds) [14: Figure III.1a-d]. The director of the Mushenge Art School pointed out to Washburn that the fact that some patterns are composed of squares and some of diamonds is merely a result of differences in the tightness of the weave [14:33]. Both this kind of variation in grid shape as well as the intermittent production of "mistakes" described above probably reflect the relative degree of patternmaker expertise rather than any intended cultural statement.

Figure 4a: Bakuba carved cup. The angles in the design appear to be right angles.

Figure 4b: Bakuba carved cup (D. K. Washburn collection.) . Same pattern as in 4a,
but the carver has made a slight affine distortion. (D. K.Washburn collection.)
Alternatively, it is possible that the variation in layout and grid is intentional on the part of the artists who are using it deliberately to communicate cultural information. For example, identical motifs reconfigured in different symmetries could be used to differentiate patterns made for in-group use from those made for distribution outside the culture. Washburn [13] found that the Yurok, Karok, and Hupa basket makers of northern California consistently used two symmetries to construct designs on basket hats they wore to their ceremonies, but reworked the same motifs into designs repeated by other symmetries on hats made to sell to tourists. Likewise, the presence or absence of "mistakes" can distinguish status groups. Among the Bakuba Washburn [14:108] found that the patterns made for the ruling Bushong were perfectly rendered symmetrical versions, whereas the versions of the same named patterns made by tribute Bakuba tribes (Bagende, Bangongo, Benapiang, Bashoba, and Bakete) contained irregularities. Other peoples, such as the Bamana tribe in Mali, insert "mistakes" into patterns on mud cloth to code information and promote ambiguities in order to show respect for elders who have greater access to knowledge and the power that comes from it [2].
Our point here is that while some peoples use mistakes to communicate cultural information, the Bakuba do not appear to embed information in mistakes, but rather in the overall nature of the pattern and in viewers’ perceptual response to it. That is, the Bakuba creatively manipulate contrasts in color, line, and texture that, in effect, produce many different patterns with simple figure/ground reversals. Thus, their focus is not on producing patterns that rigidly adhere to a rectangular grid or on producing patterns that have perfect symmetry but rather on exploiting the potential of figure/ground reversals to create different patterns without changing the underlying symmetry.
For these reasons, our analysis of this figure/ground phenomenon will examine and compare the two table patterns as if they were produced in rectangular grids. Thus, we have manipulated the skewed Mamanye pattern in Figure 1 with the help of Photoshop© software to appear with right angles as in Figure 1a, and likewise, we have manipulated the skewed Mikobi Ngoma pattern in Figure 2 to appear with right angles as in Figure 2a. In these revised states, the Mamanye pattern in Figure 1a has p4g symmetry and the Mikobi Ngoma pattern in Figure 2a has pgg symmetry, except for the "mistakes" mentioned earlier.

Figure 1a: The same Mamanye tabletop photo as in Figure 1, after an (electronic) affine
transformation has made its angles right angles. The pattern type is now definitely p4g.

Figure 2a: The same Mokobi Ngoma tabletop photo as in Figure 2, after an (electronic)
affine transformation has made its angles right angles. The pattern type is now definitely pgg.
In essence, patternmakers manipulate what is seen as figure and what is seen as ground by juxtaposing contrasting colors, lines and textures. That is, the same grid is used to generate a number of patterns that not only appear to be different but also, after the manipulations are applied, must now be described by different two-dimensional symmetry classes. Comparison of the patterns on the two table tops shows that the pattern in Figure 1a is composed of fourfold units that are created in the plain background, whereas when the same fourfold units in Figure 2a are carved, their extensions intersect in twofold over-under fashion that changes the symmetry to pgg. Algebraically speaking, the group pgg is a subgroup of index two in the larger group p4g; it is the over-under aspect of the carving that reduces the symmetry of the pattern from p4g to pgg. To show the p4g structure more clearly we have created a computer version of the Mikobi Ngoma pattern on a rectangular grid (Figure 5) where the over-under aspect is missing.
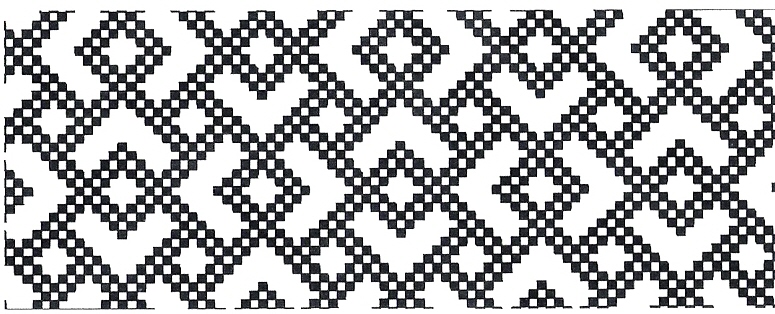
Figure 5: Computerized version of Mikobi Ngoma pattern on a Bakuba wall mat, after [12:Fig. 168].
Wall mat patterns typically exhibit no "over-under" features. As in the carved tables, not all angles
in the original are right angles. They have been made so in this computer version to emphasize the p4g structure. (Drawn by Slavik Jablan.)
Finally, it is important to note that Bakuba patterns are applied to objects with a specific orientation―they are often aligned 45º to the linear axis of the object. These contrast with patterns such as the computer-generated patterns in Figure 6 that are displayed with their axes perpendicular or parallel to the edge of the object. Mamanye patterns are never laid out in this orientation on the lid of a box or around a cup; they are always oriented diagonally to the linear axis of the piece. Although this aspect is not investigated in this paper, we suggest that there may be a perceptually-driven cultural reason for this orientation preference.

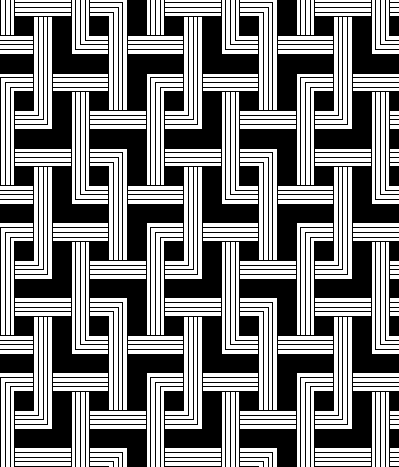
Figure 6: Computer versions of typical patterns. Left: Mamanye (p4g).
Right : Mikobi Ngoma (pgg). In practice the usual Bakuba orientation for each of these is rotated by 45º. (Drawn by Slavik Jablan.)
3. Perceptual Perspectives
It is thought that the processing of visual information occurs both at the local level of perception where separate basic shapes such as lines and dots are grouped and segregated as well as at the higher level where memory is accessed and shapes are cognitively recognized and identified (cf. [1]). The eminent visual scientist, Bela Julesz, has suggested that these two processing systems evolved to address the demands of animal perception, including that of humans, that separates things perceived into "figure" and "ground" [6:43]. It is generally easy to determine which is figure and which is ground. Kanizsa and Gerbino [7] have shown that convex contours are more readily seen as figure, and more recently, Peterson and Gibson [8,9] have argued that these contours are accessed from experiences stored in memory. There are, however, images where the figure and ground are the same shape. In these cases the distinction between figure and ground is not clear, unless either the figure or the ground is enhanced by some other feature. For Bakuba patterns the feature that captures the viewer’s attention and leads him to see one shape as the figure and another as the ground is the textural contrast between plain and carved areas or between plain, braided and plush areas.
Furthermore, because this figure/ground distinction requires the activation of global processing systems, the viewer’s past experience with and knowledge about these shapes—that is, his culture—is part of the cognitive processing of the perceived material. Because Westerners typically see pattern as something superimposed on a surface (in this case, carved into the surface of the wood) they would differentiate the patterns by the carved motif arrangements. However, it is important to recognize that this perceptual convention may not be shared by other cultures. In fact, cultural preferences and particularly artistic experiences may lead individuals to see the figure in the ground. For example, the Hopi silversmith Emory Sekaquaptewa makes overlay jewelry in which the "design", that is, the figure, is actually the cut-out shape created visually when this negative space is laid on top of a background.
The point is not whether the Bakuba see pattern in figure or in ground, but that they explicitly manipulate aspects of pattern, such as texture, that enables the creation of many differently appearing patterns on the same symmetrical base. Franquemont and Franquemont [4] have characterized a similar kind of variation in the textile designs woven by the weavers of Chinchero, Peru as a kind of improvisational "jazz."
Returning to our two tabletop patterns that are figure/ground reversals of each other, from a mathematical perspective, ignoring the over/under intertwining of the carved lines, these two Bakuba patterns have identical symmetrical grids. Indeed, for the Bakuba, two-thirds of their designs are generated by only a few symmetry types [14: Table V.2]. While the cultural reasons for these symmetry preferences have not yet been fully explored, research among other cultures [15] reveals that the preferred symmetrical arrangements are metaphors of the most important principles of cosmology and social relationships in a culture. Thus the geometric perspective reveals to us the underlying dominance of a very few pattern layouts for a large number of visually different Bakuba patterns.
In contrast, the perceptual perspective highlights how textural difference cues enable us to differentiate different patterns that have the same base layout. A perceptual perspective reveals that textural manipulations highlight different figure/ground reversals producing two variations on the pgg pattern, the p4g Mamanye pattern and the pgg Mikobi Ngoma pattern. The prevalence of such figure/ground play in Bakuba decorative pattern suggests that this kind of image manipulation is an important part of the Bakuba aesthetic system that may have important metaphoric and symbolic roles.
References
[1] Biederman, I., Recognition by Components: a theory of human image understanding. Psychological Review 94:115-147, 1987.
[2] Brett-Smith, Sarah Catharine, Speech Made Visible: the irregular as a system of meaning. Empirical Studies of the Arts 2:127-147, 1984.
[3] Crowe, Donald W., The Geometry of African Art Part I, Bakuba Art. The Journal of Geometry 1:169-182, 1971.
[4] Franquemont, Ed, and Christine Franquemont, Tanka, Chongo, Kutij: Structure of the world through cloth. In Symmetry Comes of Age: the Role of Pattern in Culture, Dorothy Washburn and Donald Crowe, eds. University of Washington Press, Seattle, pp. 177-214, 2004.
[5] Hagen, Margaret A., Varieties of Realism: Geometries of Representational Art, Cambridge University Press, Cambridge, 1986.
[6] Julesz, Bela, Experiments in the Visual Perception of Texture. Scientific American 232(4):34-43, 1975.
[7] Kanizsa, Gaetano and Walter Gerbino, Convexity and Symmetry in Figure-Ground Organization. In Vision and Artifact, Mary Henle, ed. Springer Pub., New York. Pp. 25-32, 1976.
[8] Peterson, Mary A. and Bradley S. Gibson, Must Figure-Ground Organization precede object recognition? Psychological Science 5(5):253-259, 1994a.
[9]____, Object recognition contributions to figure-ground organization: operations on outlines and subjective contours. Perception & Psychophysics 56(5):551-564, 1994b.
[10] Sekaquaptewa, Emory and Dorothy Washburn, They Go Along Singing: Reconstructing the Hopi Past from Ritual Metaphors in song and ceramic design. American Antiquity 69:457-486, 2004.
[11] Torday, E., Camp and Tramp in African Wilds. Seeley, Service & Co. Limited, London, 1913.
[12] Torday, E. and T.A. Joyce, Notes Ethnographiques sur les peuples communement appeles Bakuba, ainsi que sur les peuplades apparentées. - Les Bushongo. Annales, Serie 3, Ethnographie, Anthropologie. Documents Ethnographiques concernant les populations du Congo Belge, Tome II, Fascicule I, Musee Royal du Congo Belge, Bruxelles, 1910.
[13] Washburn, Dorothy K., Symmetry Analysis of Yurok, Karok, and Hupa Indian Basket Designs. Empirical Studies of the Arts 4:19-45, 1986.
[14]____, Style, Classification and Ethnicity: Design Categories on Bakuba Raffia Cloth. Transactions of the American Philosophical Society, Vol. 80, Pt. 3.
[15] ____, Perceptual Anthropology: the Cultural Salience of Symmetry. American Anthropologist 101(3):547-562, 1999.
[16] Washburn, Dorothy K. and Donald W. Crowe, Symmetries of Culture: Theory and Practice of Plane Pattern Analysis. University of Washington Press, Seattle, 1988.
[17] ____, eds. Symmetry Comes of Age: the Role of Pattern in Culture. University of Washington Press, Seattle, 2004.
Acknowledgments
The authors thank Slavik Jablan for the computer drawings in Figures 5 and 6, Anne Washburn for most of the photographs, and Ray Gesteland for his time-saving help in compiling the final version.